I have just finished presenting the work of this year’s Abel Prize winner, who is Pierre Deligne. In due course, the talk will appear on the Abel Prize website. As in the last two years, I have also prepared a written version of the talk, which goes into more detail. However, even the written version leaves a lot out. It was intended for a general — that is, not necessarily mathematical — audience, though I had to assume at least some maths. If your level of mathematical experience means that you find it too elementary, then I have three recommendations for further reading. I found these slides of Kumar Murty about Ramanujan’s tau function helpful and interesting. I also very much like Brian Osserman’s article on the Weil conjectures, written for the Princeton Companion to Mathematics. Finally, Nick Katz did the laudatio for Deligne’s Fields Medal and wrote an excellent article on his work. (Another article that I stumbled on only recently that looks incredibly nice, which is not about Deligne, though it mentions him, but which sheds interesting light on some of Deligne’s work is Finding Meaning in Error Terms, by Barry Mazur. So far I have just skimmed through some of it, but I think I’ll be going back to read it in more detail.)
- Comment
- Reblog
-
Subscribe
Subscribed
Already have a WordPress.com account? Log in now.
Gowers's Weblog 
March 20, 2013 at 12:22 pm |
[…] obviously can’t beat the commentary from Tim Gowers who once again spoke at the announcement about what the achievement means, so see his blog if you […]
March 20, 2013 at 12:54 pm |
Nick Katz’s article is also available at this address:
Click to access icm1978.1.0047.0052.ocr.pdf
Other beautiful surveys by Katz are:
— An overview of Deligne’s proof of the Riemann hypothesis for varieties over finite fields ;
— An overview of Deligne’s work on Hilbert’s twenty-first problem,
in “Mathematical developments arising from Hilbert problems” (AMS, PSPM, XXVIII).
Fabrice Orgogozo.
March 20, 2013 at 3:45 pm
Thanks for that — I’ve changed the link to the one you suggest.
March 20, 2013 at 1:09 pm |
[…] in current research on amplitudes. An excellent choice, congratulations to him!. Update: See Tim Gower’s blog for more, including his talk presenting Deligne’s […]
March 20, 2013 at 2:25 pm |
[…] Timothy Gowers and Arne B. Sletsjøe, are available on the Abel Prize 2013 webpage. Tim Gowers has posted on his blog some more links to background reading and said that a video of the talk he gave at the award […]
March 20, 2013 at 5:23 pm |
The situation when the same person presents the winner of a major prize 3 times in a row, is unprecedented. Could you explain how this happened? You were utterly unqualified to speak about Milnor’s work (as can be seen from your text and your own admissions on the web). I doubt that you are qualified to speak about Deligne’s work either (I admit that I read only the 1st page of your text; I will read further). Would the presenters be selected objectively, your name would never show up at all.
March 20, 2013 at 6:37 pm
Actually, the presenter of the Abel prize work is selected by the committee before the choice of prize laureate is itself decided, in order to avoid the identity of the presenter yielding clues as to the identity of the laureate, and also because the initial presentation is intended to be broadly accessible to all mathematicians and even to some non-mathematicians. So the presenter has to be capable of explaining mathematics to a general audience that may not be in his or her own area of specialisation.
Of course, the committee also prepares a number of other, more technical, descriptions of the laureate’s work, including the formal citation that is read out by the committee chairperson at the same event as the initial presentation.
March 20, 2013 at 11:15 pm
That is an incredibly rude, spiteful and disrespectful reply Mr. “sowa”.
Your crude and ill-informed insinuations might even be actionable as libel in the UK. You ought to be ashamed of yourself.
Please be advised that Prof. Gowers is a man held in the highest respect in numerous scientific and mathematical circles. I am proud that I share a planet with intellects like Prof. Gowers and Dr. Deligne.
I (and no doubt many others) found his write-up crystal clear, informative and a pleasure to read.
***Please excuse my comment, Prof. Gowers. I feel that internet “trolls” of this kind should be reminded in no uncertain terms that their toxic behavior and abuse of the Internet’s anonymity is odious to decent humans.
March 21, 2013 at 3:47 pm
sowa, what are your qualifications that would make anybody take your statements seriously?
March 20, 2013 at 8:07 pm |
Can someone who has been on the committee confirm that Grothendieck is not considered for this prize? Are only those who are likely to accept or active in the community considered? This has probably been asked and answered many times but while I am able to find several versions of this question I have not been able to find a definitive answer online.
March 20, 2013 at 8:41 pm |
To Terence Tao:
It would be nice to have this policy (and others) published by the Norwegian Academy.
This policy is so misguided that I am going have a very hard time trying to believe that it exists (I am not saying that you are trying to mislead me, just that it is extremely hard to believe that somebody even suggested such a stupid idea). To have a presenter who needs to consult the mathoverflow community (T. Gowers preparing his talk about Milnor’s work) is not a good idea, especially if the talk is for the general audience (experts don’t need these talks anyhow).
The winner is obviously known some time before the announcement, so there is time to invite an expert who is also a good speaker.
But the existence of such a policy is not a satisfactory answer to my concerns. T. Gowers is a good speaker (I know this firsthand) but not the only good speaker. There are hundreds of mathematician who understand Milnor’s works and are able to give a good or even excellent talk about them. Choosing T. Gowers 3 times in a row still calls for an explanation. It is quite clear that he is not a universal mathematician, so, no matter who the winners are, he may succeed no more than 1 time out of 3 (or 4,5, etc. if this tradition is going to continue). A success for me is not just some pleasant (and misleading) feeling of understanding in the audience.
March 20, 2013 at 9:05 pm |
To Anonymous:
I was told by a very well informed person (I don’t have any permission to mention his or her name) that the Norwegian Academy wants as a winner somebody who will attend the ceremony, talk to the King, etc. This cut off some obvious candidates, like H. Cartan, who was over 100 and would not be able to attend simply due to his very advanced age (he passed away since then).
Since there is no doubt that A. Grothendick will not come, and, moreover, will decline the prize (as he did with a Swedish prize in 80ies), he is not a candidate. Of course, he is the best living mathematician, and such consideration should be never taken into account. As a famous recent example, one may mention G. Perelman, who clearly stated that he will decline the Fields medal if awarded, and was awarded despite this.
Last year the Abel prize committee missed a rare chance to award prize to one of the best mathematicians of all times, and at the same time to do something good for a dying person. I mean W. Thurston, probably the best geometer of all times (by which I mean a mathematician with strongest visual thinking, and not just being formally classified as a geometer). He was ill, and the nature of his illness was such that his chances to survive another year were approximately zero. He died in the last August.
Instead of this, the prize was used to promote T. Gowers tastes.
March 20, 2013 at 9:18 pm
It is completely unacceptable to write comments like yours anonymously. It’s obvious that you deeply hate Gowers, but publicly spreading unsubstantiated insinuations like “instead of this, the prize was used to promote T. Gowers tastes” under a pseudonym is beneath the level of a civilized discussion. If you want to fill the comment section with anti-Gowers hatespeak, at least do it under your real name.
March 20, 2013 at 9:44 pm |
good choice, Abel is also very strong in street fighter
March 20, 2013 at 9:51 pm |
@sowa I think you missed the point that Terry made, which is that if an expert in the relevant area is chosen, then when that expert arrives in Norway it immediately gives a big clue about who the winner is.
As for your statement that the prize was used to promote my tastes, there is a sense in which it is true and a reading-between-the-lines sense in which it is false. The true sense is that the prize was awarded to a mathematician whose mathematics is very much to my taste (though I wouldn’t want to suggest that the work of mathematicians such as Milnor and Deligne is not to my taste — it’s just a lot further from things I know about), and if you award the prize to somebody, then to some extent you promote their area of mathematics. The false sense is the suggestion that I had any influence whatsoever over the decision.
Incidentally, you may be reassured to know that in May, the day after the prize is presented by King Harald of Norway, there is a one-day symposium on the work of the prize winner. That will feature talks by experts, as it does each year.
March 20, 2013 at 11:30 pm |
To Timothy Gowers:
No, I did not missed this point. This argument is appears to me to be so stretched that I still hesitate to refute it. But it seems that I have no other choice.
First, I never understood why this secrecy is needed. Anyhow, any clues are irrelvant. One can announce the winner as soon as it was selected, and to have a formal presentation and the ceremony later, for example. If by some reason some people want both things happen at the same day, the presenter may arrive to Norway one hour before the ceremony. I doubt that this will give clues to anybody who does not knows this already. If the presenter is invited from overseas, she or he may travel first to some European country. Do you think that the arrival of some mathematician to Paris will be clue? Definitely, there are many other options. Other prizes are awarded without such a policy, and manage not only to keep, but to increase their prestige.
On the other hand, you missed one of my points in my reply to T. Tao. Namely, even if this argument is accepted, the fact that you are the presenter 3 times in a row calls for an explanation.
I fail to understand how work of a mathematician can be to somebody’s taste if that person has no idea even about the most famous work of this mathematician.
Definitely, you will disagree, in contrast with many other mathematician, that the level of E. Szemerédi is not even close to the level of Milnor or Deligne. As a crude estimate, I would say that there are about 2 orders of magnitude more mathematicians of the level of E. Szemerédi than of level of Milnor or Deligne. Given this, I cannot believe that the decision to award prize to E. Szemerédi was arrived on purely mathematical grounds. Since you are the main and for few years the only promoter of E. Szemerédi work during the last 15 years (earlier were none), the natural conclusion is that you did used your influence.
I have no idea why I should be “reassured” by another ceremony in May. I do not need any talks about the work of Milnor, E. Szemerédi, or Deligne – they will add nothing to my knowledge.
March 20, 2013 at 11:36 pm
“the fact that you are the presenter 3 times in a row calls for an explanation.”
I did it once, they were happy with the job I did, so they asked me again, twice. That is the explanation. Also, I am not the first person to have done it more than once.
“Since you are the main and for few years the only promoter of E. Szemerédi work during the last 15 years (earlier were none),”
That is complete nonsense.
“the natural conclusion is that you did used your influence.”
The conclusion may come naturally to you, but it is nevertheless false (and, as I’ve just said, based on a false premise anyway).
March 21, 2013 at 12:18 am
To Timothy Gowers:
Sorry, I do not buy this explanation.
Who else did 3 presentations in a row? Of course, I may read their whole website, but it seems that you just know.
The statement “That is complete nonsense” does not proves or refutes anything. I am trying to present at least some reasons for my position and my conclusions, but your replies are just “nonsense”, “false”. Sorry, this is not just unconvincing, but actually almost completely eliminates any doubts for me.
March 21, 2013 at 1:35 am
Marcus du Sautoy presented the Abel laureates work in 2006, 2008, and 2010.
March 21, 2013 at 2:18 am
To T. Tao:
I failed to find anything by M. du Sautoy at their site, although I do remember him talking about the work of Thompson and Tits, most likely. Did he really presented the work of Lennart Carleson in 2006?
Anyhow, this wouldn’t be 3 times in a row, and if he spoke in 2008 and 2010, he would be talking about his field of expertise. Most importantly, he doesn’t has such influence, so this is of almost no importance.
March 21, 2013 at 2:36 am
The announcement of du Sautoy presenting Carleson’s work can be found at http://www.abelprize.no/nyheter/vis.html?tid=46169 , though unfortunately the video link seems to be broken.
I think you may have an inaccurate impression of the significance of the role of presenter of the laureate’s work; it is part of the general outreach aspect of the Abel prize (as are the Abel lectures, or the popular descriptions of the laureate’s work which for the last few years have been written by Arne Sletsjøe), but is not connected at all with the decision making process of the Abel prize committee, except that the committee asks the presenter if he or she is willing to come to Oslo to give the presentation before any decision on the prize laureate is made.
March 21, 2013 at 4:55 am
To T. Tao:
The video link is indeed broken and leads to this year front page, but the text at your link convinces me that I was wrong. Well, this strange choice of the presenter of works of Carleson adds to the rapidly growing collection of mysteries surrounding the Abel prize.
For me, the decision making process is a black box, and the only way to guess anything about it is to observe the output of this black box. The secrecy surronding all mathematical prizes only undermines their credibility. The Abel prize fared relatively well, especially if compared to the Fields medals with their bizarre age restriction and secret committee. Still, the failure to award Abel prize to H. Cartan, I.M. Gelfand, and W. Thurston is not excusable. As is the award of the prize to E. Szemerédi.
The significance of the presenter is negligible if she or he is a professor at an obscure Norwegian university. It is not if he is a Fields medalist and a FRS. In the last case he is the face of the prize for the general public, which has the last word in the questions like support of various branches of mathematics, creating or closing the mathematics departments, etc. Marcus de Sautoy is somewhere in between. Definitely, presenting the work of Milnor and Deligne will increase Gowers’s influence (and many will think that he indeed understands their work). And it is going to be very hard to convince me that the choice of Gowers’s is purely accidental and he made no efforts to get invited.
If this issue really bothers you, you can do a couple of things which will convince me (and others less inclined to write something online even under a nickname). First, announce the next year presenter within 2 months, and post this announcement on the prize site. Initially I wrote two suggestions, but then deleted the second. I am sure that you will be able to guess it.
March 21, 2013 at 12:37 pm
“And it is going to be very hard to convince me that the choice of Gowers’s is purely accidental and he made no efforts to get invited.”
Given your attitude, I’m sure that’s true. But it is a fact (for which I cannot offer a proof, but note that when I dismissed another statement of yours as nonsense and didn’t offer a proof, I was still correct — in that case Van Vu helpfully provided the proof) that until I was asked whether I would present the work of the 2011 winner, I did not even know that there was a special announcement ceremony for the Abel Prize. When I was asked, I was told that I should not worry if the winner was from a very different area from mine: in general the organizers regarded that as almost an advantage, since it meant that the presenter would be more in sympathy with the audience of non-mathematicians. Incidentally, I think I’ve been fairly public about the fact that my understanding of Milnor’s and Deligne’s work (and even to some of Szemerédi’s work when it comes to it) is extremely limited. But that was sufficient for a general-audience talk.
One final remark, since I think this discussion has gone on long enough: you refer frequently to some kind of “policy” for choosing the presenter. But I don’t think there is a policy: they are just looking for someone to do a certain job.
March 22, 2013 at 12:52 am
To T. Gowers:
I did not notice that Van Vu provided a proof. I will not repeat myself, I replied to him.
Of course, you were quite open about your level of understanding of Milnor’s and Deligne’s work. I do appreciate this a lot. My whole picture is based entirely on your own words. A mathematician may knew a branch of mathematics relatively well without ever using it in her or his work (a rare situation nowadays, of course). But only very limited number of mathematician will search the web for the relevant posts by you; the very possibility that you asked for help on mathoverflow will never cross the mind of anybody who is not following your activities systematically. It did not cross my mind either; I read about this (in your blog, if I remember correctly) by a pure accident. I did read your text posted on the prize site; my reaction was: of course, the description of Milnor’s work is utterly inadequate, but what one can expect when speaker works on the opposite side of mathematics, what a strange choice.
It seems that I need to reiterate what policy I am taking about. It is the policy of selecting the presenter before the winner is determined. I was told only one reason for this: not to provide a clue. I suggested a few ways to address this concern without raising any suspicions about the integrity of the whole process.
March 21, 2013 at 12:07 am |
To Michał Kotowski and postdoc:
I encountered such accusations before. People use them when they are unable to refute the arguments on substantive grounds.
Especially amuzing to hear such accusations from people who sign a “Anonymous” (not here), or “postdoc”.
Dear postdoc, your nickname tells absolutely nothing about you. My nickname, “sowa”, is known to many people and some do know my real life identity. I decide on my own to whom tell about it, and to whom not.
Apparenty, you believe that even completely anonymous comments are allowed here if one expresses an admiration to the author of this blog, but no semi-anonymous criticism is allowed.
I am well aware that respect T. Gowers commands (this is clear even from my comments to this post); you don’t need to tell me about this. Actually, if not this respect, I wouldn’t bother to write here. I cannot respond to everything wrong happening or reported at the web.
Dear Michał Kotowski and postdoc,
Could you tell me what you would gain if I would use my real life name? You definitely don’t know me personally. What kind of information you expect to get from my real name?
Actually, it is exactly the persons like you who lead to my relative anonymity on the web. When I started a blog at some other sevice almost ten years ago, I thought that every mathematician in that community will immediately figure out my identity. Some did, some failed, but only malicious persons attempted to use it. Some especially nice people simply refused to try find out my real life identity. Such people were among the first whom I told it.
Before your comments I planned to made some changes to one of my websites which would directly connect the nickname “sowa” with my real life identity.
Thank you for reminding me that this is a rather doubtful way to go.
March 21, 2013 at 12:30 am |
To the host of this blog: I don’t know what comment moderation policy is usually employed here, but I’d suggest deleting all “discussion” with user sowa, since it’s clear it amounts to nothing but trolling and doesn’t add any value.
March 21, 2013 at 12:50 pm
I’m very reluctant to delete comments, apart from obvious spam. That applies particularly to critical comments. I would feel very awkward about censoring them — I’d rather leave them up and let people judge for themselves whether they are justified. I think sowa is basically well-meaning and entitled to his/her opinions, even if he/she chooses to express them in an unusual way.
All that said, I won’t be sorry when this particular discussion peters out.
March 21, 2013 at 12:57 am |
Dear sowa
You wrote:
“As a crude estimate, I would say that there are about 2 orders of magnitude more mathematicians of the level of E. Szemerédi than of level of Milnor or Deligne.”
I do not think anyone would qualify to make this kind of statement. It is already hard to have a deep understanding of all important results of each of these mathematicians, let along all
three.
About Szemeredi, he has lots of very influential works (not only the
arithmetic progression theorem), and each came with highly original ideas, never seen before. Many of these ideas become
standard tools with hundred of applications.
Your accusation that Tim is the only one who advocates Szemeredi’s work in the last 10-15 years is false.
Beside Tim, there are many mathematicians of the highest caliber, use (and naturally advocate) his mathematics.
As an example, few years ago, IAS run a whole semester program
on arithmetic combinatorics, focusing on expanders and growth in groups (things people like Lubotzky usually study). The whole development here was influenced by Szemeredi’s work on sum-product phenomenon and incidence problems in geometry.
March 21, 2013 at 2:10 am |
To Van Vu:
You misinterpreted my words. Perhaps, I should write in more details, but these comments are too long already.
Wide mathematical public learned about the Szemerédi’s theorem from the work of Furstenberg, who provided a conceptual approach to it. Various extensions of Furstenberg’s results turned into a small branch of the theory of dynamical system, followed only by few. Then T. Gowers’s gave a talk about the theorem at the 1998 Congress. A lot of people there were quite diappointed; they expected him to speak about his work on Banach spaces. (I wasn’t there, but some my close friends did attended 1998 Congress.) When I wrote about few years, I meant 1998 and the next few years. In early 2000’s the topic turned to be relatively popular. The real interest was stimulated by 2004 Green-Tao work, which a lot of people (including myself) mistook for a work about prime numbers.
Expanders and growth of groups (in various versions) go back to the works of Margulis, Milnor and Gromov.
Why nobody would qualify? To have a full command of methods of these 3 mathematician (why only these 3? – the are other Abel prize winners of the same level as Milnor or Deligne) is hardly possible nowadays, when mathematicians are excpected to produce several publications a year and are employeed as overqualified instructors of meaningless courses. There is simply not enough time. But it is not hard to understand the most famous results, and for Milnor and Deligne – why they are important. As of Szemeredi theorem, I fail to understand why it is interesting at all. Even the presentation of T. Gowers himself (see p.5 of the text on the Abel prize site) does not help. Basically, he argues that it is important because it has simple statement and difficult proof. (But Furtenberg’s proofs is in no way as intimidating as the original one.) And one can study some parts of the work of tthese people in some depth. For example, it is not difficult at all to understand the proof of Szemeredi’s regularity lemma – apparently, the most useful part of his proof.
P.S. I don’t know why, but I do not get comments in e-mail. So, it is already fairly difficult for me to follow this discussion. I apologize in advance if my replies will be not timely.
March 21, 2013 at 3:22 am
@Sowa
I usually feel unqualified to comment on Tim’s blog. But it seems to me from your comments that you have a low opinion of (pure) combinatorics and the likes and little more, since you seem to be suggesting that the theorems were not a big deal and the proofs no big deal either and hence what was the big deal anyway? And since you are commenting anonymously I am surprised that such comments are even getting published. An article by Terence Tao specifically talks of the influence Szemeredi’s Theorem (just the arithmetic progressions theorem in specific, Szemeredi has had an extraordinary range and all that does not even get mentioned) has had in a number of areas of Mathematics http://arxiv.org/pdf/math/0702396v1.pdf I feel that this is actually quite extraordinary (and note that even the Regularity Lemma is basically barely mentioned). The Regularity Lemma can deserve a similar document since it is not just a lemma but almost a philosophy indicating deep connections between several areas of Mathematics (the same probably holds for many of his results, such as in incidence geometry).
March 21, 2013 at 5:21 am
To Shubhendu Trivedi:
Wow! You raised extremely intresting and important questions in your comment. The comments to this post is hardly a suitable place for disussion of these issues. You are correct, I think that theorem are not a very big deal. I agree with a maxim attributed to Yu. I. Manin: proofs are more important than theorems, and definitions are more important than proofs. I prefer to the Tao’s paper, which I am, of course, familiar with, a much shorter article of S. MacLane – it is only 2 or 3 pages long. It is well known that S. MacLane, whom I consider as one of best mathematicians of the previous century (and yes, his main contributions are deep definitions and not difficult to prove theorems) had a very low opinion about “Hungarian combinatorics”. MacLanes ideas changed many areas of mathematics and later even of theoretical physics. In contrast, I am aware only of applications of Szemerédi’s theorem to prove similar theorems, similarly strange and useless. Well, I have to stop here – this is an infinite topic.
March 22, 2013 at 9:38 pm
While it is true that the early development of expander graphs (by Margulis, Lubotzky-Phillips-Sarnak, etc.) relied primarily on methods from geometric group theory and number theory, the modern developments in the subject (as described for instance in this survey of Lubotzky) are much more combinatorial in nature, and have indeed been strongly influenced through the work of Erdos and Szemeredi (through their sum-product theorem), as well as parallel work of Wigderson et al. on the zig-zag product construction of expanders; these are the recent developments that Van is referring to. As in other areas of mathematics, the combinatorial arguments are good at handling “unstructured” cases, while the more algebraic arguments are good at handing “structured” cases, and often it is a combination of the two methods that is ultimately needed to get satisfactory results. For instance, to demonstrate expansion in Cayley graphs for the special linear groups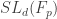
over a finite field of prime order, the original arguments of Margulis are applicable when 
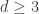and the generators are fixed, basically because 
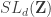obeys Kazhdan’s property (T) in this case. But when d=2, property (T) is not available. Selberg’s 3/16 theorem on the spectral gap on the Laplacian on arithmetic hyperbolic manifolds can then be used as a substitute when the generators, when lifted up to the integers, generate a bounded index subgroup of 
, but it was an open problem (Lubotzky’s “1-2-3 conjecture”) for a long time as to what happened for much thinner subgroups of 
. This was eventually settled using primarily combinatorial means by Bourgain and Gamburd, building on the work of Helfgott, which in turn built on the sum-product theorem of Bourgain, Katz, and myself, which finally originated from the sum-product result of Erdos and Szemeredi. (Lubotzky’s survey linked to above describes most of these developments.) This has led back to some further developments in geometric group theory (concerning the strong approximation property and the like), as well as to the Bourgain-Gamburd-Sarnak sieve in analytic number theory as mentioned in Matt’s post (which for instance can be used to sieve out almost primes in Appolonian circle packings).
March 22, 2013 at 9:58 pm
Incidentally, an absolutely crucial ingredient in many of the above recent developments in expander graphs is the Balog-Szemeredi-Gowers lemma, which is a deceptively simple lemma in graph theory whose original form first appears in this paper of Balog and Szemeredi, and was later improved by Gowers in his own work on Szemeredi’s theorem. It turns out that this lemma is precisely what is needed (together with the sum-product theorem dating back to Erdos and Szemeredi) by Bourgain and Gamburd in order to get the needed expansion for Cayley graphs for the middle of the three time periods required to establish mixing. (For the last time period, one needs Gowers’ theory of quasirandom groups, while for the first time period one uses more algebraic methods, e.g. the Lefschetz principle.)
March 23, 2013 at 12:56 am
To Terence Tao:
Thank you very much for the references in these comments and in the other; I had downloaded all these papers, but it is highly improbable that I will study them in any depth. The Bourgain paper looks especially forbidding. He doesn’t says a single word about what he is doing and why. Apparently, this is the case with all his papers (of course, I had seen only a fraction of them).
Let me tell how your story looks in my eyes. I am hardly interested in expander graphs for their own sake. For me, they are interesting only because the methods of the sort used by Margulis can be applied to them. I suspect that Margulis did this work only because he was formally employed by the Institute of Problems of Information Transmission (even if you are Margulis and live in Moscow, you still need to do something at least remotely related to the interests of your employer). When the connection with such abstract methods disappears, I lose interest. Similarly, for a long time I paid some attention to Szemeredi theorem only because it can be proved by methods of Furstenberg. I am back to this state, after an interruption.
When you move from SL(n) for n>2 to SL(2), you are usually change the branches of mathematics. I do not think that a theorem not dealing with the SL(2) case is in any sense incomplete. There is similarly sounding problem in another branch of mathematics, but this does not diminishes the significance of your result. SL(2,Z) is essentially free group, and, as somebody said, a free group is not a group, but just a collection of words (there is a reference for this maxim, but it is attributed there to “somebody”). Of course, by moving to SL(2,Z) you are leaving the realm of crystal-like groups and enter an amorphous area where methods of combinatorics appear to be more suitable. I do not find this to be remarkable; in fact I wrote long before this discussion that combinatorial arguments occur everywhere and often it is much easier to use them than to search for appropriate abstract context.
I do not see any reason to believe that the theory of expander graphs is intrinsically related to Margulis-like methods or to modular forms. It is quite remarkable that a connection exists, but, apparently, expander graphs are returned to their proper realm of analysis and combinatorics.
March 21, 2013 at 3:20 am |
Wow, someone has an ax to grind here. I find statements such as “As a crude estimate, I would say that there are about 2 orders of magnitude more mathematicians of the level of E. Szemerédi than of level of Milnor or Deligne” to be extremely distasteful. It reminds me of the discourse of grade school kids. Anyway, it would have been nice if sowa had spent all that energy on enlightening us about Deligne rather than completely hijacking the discussion and turning it into a very unpleasant read.
March 21, 2013 at 3:42 am |
@SOWA: If you are not satisfied with TG’s past performances, I suggest you contact the Abel Prize committee so that they can either,
(a) change the rules so that experts can be asked to present the laureate’s work, or
(b) nominate another candidate that is deemed more competent to present next year’s work.
There is no need to attack TG for the Abel prize’s policy. The committee simply asked TG whether he would be willing to it, and he kindly obliged.
Finally, I do not see why TG’s past appreciation (“promoting”, if you will) of Szemeredi’s work is relevant at all. Are you perhaps suggesting that TG started planning the manipulation of last year’s Abel prize more than a decade ago? Or did he use a time machine instead?
I suggest you formulate your thoughts more clearly.
March 21, 2013 at 5:31 am |
To Richard Séguin:
Don’t you think that it is your discourse wich is at the level of kindergarden? Only curses and no substantive arguments. I cannot hijack this or any other discussion because nobody is obliged to read my comments, much less to reply to them.
There is not enough space here to enlighten you about Deligne works. In fact, there are good expositions and I see no point in replicating them. An in depth understanding cannot be achieved by reading comments in a blog anyhow; it requires years of study. Start with a textbook, or, better, with Grothendieck-Dieudonne EGA.
March 21, 2013 at 5:49 am |
To Wolfgang M:
Actually I did contacted the committee, right here. I suggested a less drastic change of rules than (a). As of (b), I definitely can do this if asked.
I believe that no policy alone can result in selecting the same person for 3 consecutive year.
More than decade ago there was no Abel prize; the first one was awarded in 2003. Please, do not attribute me your fantasies. Things are more mundane and more complicated than time machines. I apologize if my comments are not quite clear; I am quite sure that they are absolutely clear to the people “in the know”, in particular to T. Gowers and T. Tao, even if they will not admit it.
March 21, 2013 at 6:27 am
@SOWA:
Let us be very generous and give you the benefit of the doubt: you are trying to make this world a better place. Let us also assume that you have contacted the Abel Prize committee with some (constructive) suggestions for improvement. Good! Great! Excellent!
What more do you hope to achieve by being unusually rude and condescending on this particular blog? I honestly can’t tell because your answers are so unclear. For example, in the post above:
> What *exactly* is it you are accusing TG of and what is the *purpose* of that accusation?
I suggest that, if you really intend to become a mathematician some day, you try to express your emotions more clearly. (This is not just a language problem.) Right now it looks like you are here because you are angry and you want to damage the reputation of some individuals and you do not care about making falsifiable statements. If this is true, then I don’t know why anyone should pay any further attention to your incoherent/non-transparent posts.
March 21, 2013 at 12:35 pm
To Wolfgang M:
And why then do you pay attention to my comments?
It you who is accusing (myself), not me. I asked a specific question, then expressed some opinions. What is rude about this? I fail to understand why you are so upset by my opinions, which are quite widespread. If I am just an angry persone who cannot even write clearly, then how can I damage the reputation of anybody?
Finally, your last paragraph made me smile. I do not intend to become a mathematician, I AM A MATHEMATICIAN. I wrote some comment in this blog twice – now and one year ago, after the prize was awarded to E. Szemerédi (I had no intention, but somebody partially quoted my comment in the blog of a friend). If you fail to see from these coment that I am a mathematician, then, very likely, you are not, sorry. Good luck.
March 22, 2013 at 1:16 am
@SOWA:
You still have not given an answer to my questions, which I think are very reasonable in this context. Please do answer:
1) What is it you are accusing Gowers of? Be precise!
Has he bribed the Abel Prize committee? Blackmailed some of them? Used telepathy or a Jedi mind meld to change the outcome of the voting process? Replaced voting ballots? Deleted posts on blogs that discuss the works of mathematicians that are eligible for the Abel Prize? If your answer is: “He has mentioned the works of Szemeredi on several occasions in texts no one is forced to read,” mine is: “No one has ever disputed this and nobody cares. He has every right to do this. Anything else you can add to the conversation?”
And who are “those in the know” that are conspiring (what? against whom? how?)
2) What do you wish to accomplish by being so rude on Gower’s own blog? Be clear!
If the answer is: “I want to make sure he or other people no longer mention the work of Szemeredi, not even on a private blog,” then mine is “close this browser because that is not going to work.” Same answer if you think Gowers can take back Szemeredi’s prize and give it to a mathematician of your choice.
Finally, I did not mean to make you smile or use ALL-CAPS.
March 22, 2013 at 3:33 am
To W:
I will assume that W and Wolfgang M is the same person.
It is you who is saying that I am accusing T. Gowers in something related to the Abel prize. Please, tell me, what you consider as an accusation. I have no idea.
Please, restrict your imagination a little and do not put your words in my mouth even as a conjecture. I will not comment on your fantasies.
I did not say that people “in the know” are conspiring, and I intentionally put the quotation marks. Some people know this, some know that, and sometimes some people know more than the others. I cannot give here explanations which every user of internet will understand. I wrote this in a generic form as a reply to you generic statements that my comments are sometimes (or always) are not clear to you. In my comment these people “in the know” include me. Do you suspect that I am conspiring with T. Gowers and T. Tao?
March 22, 2013 at 5:15 am
@SOWA: If you don’t remember accusing anyone of anything, let me help you:
1. “The situation when the same person presents the winner of a major prize 3 times in a row, is unprecedented. Could you explain how this happened? […] Would the presenters be selected objectively, your name would never show up at all.”
Your claim: “Gowers was not selected objectively.” What does that even *mean*?! Do you mean “in a fair way/democratically”? *Who* was being subjective? *In which way*?!
and
2. “But the existence of such a policy is not a satisfactory answer to my concerns. […] Choosing T. Gowers 3 times in a row still calls for an explanation.”
and
3. “Namely, even if this argument is accepted, the fact that you are the presenter 3 times in a row calls for an explanation.”
and
4. “[…] I cannot believe that the decision to award prize to E. Szemerédi was arrived on purely mathematical grounds.”
*Which grounds then*?
and
5. “[…] the natural conclusion is that you did used your influence.”
*How* did Gowers influence the selection process in an *unfair*/unethical way?
and
6. “Instead of this, the prize was used to promote T. Gowers tastes.”
and
7. “Sorry, I do not buy this explanation. Who else did 3 presentations in a row? Of course, I may read their whole website, but it seems that you just know.”
Basically you are calling Gowers a liar. Tao illustrated that spending a few mins on google proves you wrong.
and
8.”And it is going to be very hard to convince me that the choice of Gowers’s is purely accidental and he made no efforts to get invited.”
Again you claim Gowers used his “powers” to force his own appointment as Abel Prize presenter?!
I count 8 distinct instances of SOWA accusing Gowers or others. If SOWA thinks that s/he has not accused anyone at all, I’d like to know which field s/he is working in. Pun intended.
March 22, 2013 at 11:35 am
To W:
Too bad. Initially I thought that I finally understood what do you mean by an “accusation”, but then noticed that only a part of your enumerated quotes fits into the conjectured pattern. So, I am still at loss.
As of your quotes and your questions, the answers are mostly already here, except that I do not know the answer to your question 5, and I find your question to be moderately interesting, but not more. I don’t know if T. Tao used Google, but he did not proved that I am wrong. Actually, he provided a new illustration of the absurdity of the whole procedure. Marcus de Sautoy is a good mathematician and a good writer, but he hardly suitable to speak about L. Carleson’s works. Even if presented Carleson’s work (this was hadr for me to believe in), only T. Gowers was a presenter at 3 consecutive awards. I wonder if he will be the presenter next year.
Apparently, you are not interested in discussing Abel prizes, mathematics, combinatorics, algebraic geometry – anything related to the post. Your main subject of interest is SOWA. I do not think that such a discussion is suitable here. Please, discuss this somewhere else.
Good luck.
March 22, 2013 at 3:39 pm
If you still deny having made any accusations, I’m outta here! That statement is just too dishonest for me.
March 21, 2013 at 5:58 am |
To all dreaming about silencing me:
My first comment was addressed to T. Gowers; I readily accept replies not from him, but from T. Tao.
But, frankly, I am tired of taking about my so-called anonymity and my lack of respect to some theorems and mathematicians. So, I will not reply anymore to complaints about my undisclosed identity and the lack of appreciation of E. Szemerédi’s theorem.
Please, keep in mind that T. Gowers is not a kid and is quite capable of replying to me, ignoring me, moderating the comments if likes, banning me from commenting, etc. This is his blog, not yours (and not my). Show some respect to him, please.
March 21, 2013 at 6:27 am |
@Sowa,
I didn’t *raise* questions. I only tried to paraphrase what you said in the comment ( that I replied to) through the lens of what I understood to be the underlying reason from your various comments. Thanks for vindicating me, even if indirectly.
You resort to “proof by call to eminent authority” to make your point a few times in your comment. Well, I won’t be able to do that and hence this won’t convince you perhaps.
And although I am admittedly unqualified to make any such claim, I have the highest respect for “Hungarian Combinatorics” having seen some of them first hand, and this is as much as I have for the more abstract mathematics that I observe my friends do and talk about. The “Hungarian Combinatorics” you mentioned _also_ changes many areas of mathematics but in an indirect sense and I think Tao’s article there tries to emphasize that precise point.
March 21, 2013 at 12:48 pm
To Shubhendu Trivedi:
I am disappointed. These questions are indeed interested and debated regularly.
I am not trying to prove anything by appealing to authorities. I refer to them as I would refer to a mathematical paper or monograph in my research paper. Why should I repeat MacLane instead of referring to his article? Why do you allow yourself to refer to a paper by Tao, but do not like when I refer to MacLane?
March 21, 2013 at 8:44 am |
[…] de los blogs que sigo también se hacen eco de la noticia, el de francisthemulenews y el de Gowers, que de hecho es el encargado de realizar la … del […]
March 21, 2013 at 2:07 pm |
sowa: I don’t think that it is constructive to make accusations in a public forum of which you yourself are not entirely convinced (how could you be? your evidence is entirely circumstantial, and as we know, correlation does not imply causality). The only effect you might hope for would be cheese off T. Gowers, and to what end?
Wouldn’t e-mail be a better medium for this exchange, if it need take place at all? (I’d think that the Abel Prize committee would actually be a more suitable address… but I also want you to post more about math, because I’m guessing I’d be very interested in any small mathematical insight you might be willing to share, if you’re whom I conjecture you are 🙂 )
March 21, 2013 at 10:15 pm
Haha, just imagined it could have been Grothendieck. Made me giggle ^^
March 22, 2013 at 2:00 am
To Anonymous:
Why your imaginations stops at Grothendieck?
March 22, 2013 at 2:42 am
To dmoskovich:
This time I got to the stage of guessing (not demanding) my identity very fast. 🙂 Would you risk writing a short e-mail to the suspect? If I will get your letter, I will be very happy. I don’t know your identity and will not try to guess it.
There are two distinct groups taking part in this discussion; you seem to be the only one so far who partially belongs to both (may be because you mistook me for being somebody else). The first group consists of T. Gowers himself, T. Tao, and V. Vu. They take my questions at their face value and try to answer them. Often I find their arguments unconvincing, but this is another issue.
Other people accuse me of making accusations. I really don’t know whom I accused here. I asked how the same person could be the presenter 3 times in a row, especially because 2 times out of 3 he had almost no idea about the things he was supposed to present to the public. May be people think that I am accusing T. Gowers in the lack of knowledge of works of Milnor and Deligne? But he himself wrote about this, and nobody is expected to study their works, except if this is needed for his or her research. I was told about a policy of the Abel foundation. I expressed the opinion that this policy is extremely stupid. OK, may be this is an accusation in stupidity. If I believe that some people are stupid, it would be quite stupid of me to appeal to them directly. The most effective way, namely to work with them behind the closed doors, is not available to me. That remains is to speak at a public forum, exactly what I am doing.
By the way, notice that T. Tao is a member of the Committee, so, in a sense, I am writing to the Committee. But as he told us, this is not the Committee policy (a Committee serves 2 years, and then a new one is selected by an unknown to the public process), this is a policy of Abel foundation.
Finally, could you tell me what you had in mind when you wrote that I write things in which I am myself “not entirely convinced” (and cannot be)?
The internet mantra “correlation does not implies causality” is rarely relevant. Correlations are important because they allow predicting things (not definitely, but with high probability, if the correlation is high). Usually, this is enough.
March 21, 2013 at 3:27 pm |
This whole discussion comes down to the point that sowa doesn’t like Hungarian-style combinatorics. Fine, if I don’t like number theory I could equally well say Deligne only proved weird statements about numbers, useful for nothing but proving similar strange and useless statements about numbers. What’s the difference? What do any of us get out of trashing other areas of mathematics?
It is not possible to defend Szemeredi or any other combinatorialist as a great mathematician to someone who rejects combinatorics as an interesting area of mathematics, so there isn’t any point trying. In this case the person doing the attacking is a conspiracy theorist (`people in the know’ and such like statements) and it’s probably better for sowa’s mental state not to provoke.
March 22, 2013 at 3:05 am
To Pete:
You see, in a precise sense all mathematics consists of complicated statements about natural numbers (and, hence is a part of number theory). But it seems that you had in mind something less tautological. Perhaps, your opinion about works of Deligne is based only on the T. Gowers presentation (this is why I so strongly object to having non-experts as presenters). His proof of the Ramanujan–Petersson conjecture is only a minor part of his work. He even did not write a detailed exposition, as he almost always does. Even the completion of the proof A. Weil’s conjectures is not his deepest contribution.
I invested a lot of time in studying the “Hungarian combinatorics”. After this, it is indeed hardly possible to change my opinion in comments to a post.
And what is my conspiracy theory? I am not aware of any related to these topics; it would be quite interesting to learn about at least the one attributed to me.
Actually, I started to write a reply not noticing the last phrase. You do not deserve any reply, but I will post what I wrote for the benefit of others.
March 21, 2013 at 6:12 pm |
There is a blog by sowa called “Stop Timothy Gowers!!!!”:
http://owl-sowa.blogspot.com/2012/05/my-affair-with-szemeredi-gowers.html
It’s quite and interesting read. I think it reinforces the idea that there are at most two kinds of mathematics by insisting that there is only one.
I wonder what sowa thinks about other areas of mathematics outside of algebraic geometry and combinatorics, for example, differential equations, functional analysis, or probability?
March 21, 2013 at 10:23 pm
Wow — that’s quite flattering!
March 22, 2013 at 3:12 am
To Timothy Gowers:
In fact, a link to the mentioned blog was posted in a comment in your blog almost a year ago. Its title was changed yesterday, and there a few words there about this, but they hardly explain this change.
March 22, 2013 at 6:41 pm
Dear sowa,
I read your blog post on the two cultures, and tried to post a comment, but it seemed to require a sign-up which I was too impatient to complete. So let me post my comment here (where it may also gain more exposure).
Both in your post, and in a comment above, you state that the Green–Tao theorem is not really about prime numbers. This was something noted by several people upon the appearance of their first paper, but it is not really relevant (as far as I can see) in light of their subsequent work, in which they get precise asymptotics for many kinds of linear equations in primes, by mixing additive combinatorics/dynamical systems concepts such as Gowers norms and nilsystems with classical topics of analytic number theory such as the Mobius function.
One could also note the role that additive combinatorics concepts such as the sum-product theorem, and its applications to proving the expander property for certain Cayley graphs associated to finite groups of Lie type, have played in other developments in analytic number theory too, such as the Bourgain–Gamburd–Sarnak sieve.
I think it is interesting that topics from the “second” (supposedly non-structured) culture are actually supplying structural perspectives on analytic number theory (surely a “first culture” subject). I’m not very convinced about the distinction between the two cultures in any case, and this example of the recent interaction between additive combinatorics and analytic number theory is one of the reasons why.
Regards,
Matthew Emerton
March 22, 2013 at 8:35 pm
I might add that the topic of estimating polynomial exponential sums (i.e. Mordell sums)
is one interesting place (which, among other things, is of importance in cryptography) where the contributions of the two most recent laureates Deligne and Szemeredi almost overlap and can be directly compared, as is done for instance in this article of Bourgain. Roughly speaking, the Weil conjectures allow one to control this sum adequately in the “structured” case when the polynomial 
have low degree, but do not give satisfactory bounds in large degree even if one uses the full strength of Deligne’s work. However, Bourgain and his coauthors have shown that the sum-product theorem (which originates in this paper of Erdos and Szemeredi, although it has been heavily developed since then) allows one to nontrivially bound this sum in the complementary “unstructured” case when the degree is allowed to be large (but the polynomial is sparse in a certain technical case); among other things, Bourgain and Konyagin were able to obtain for the first time equidistribution results for sparse multiplicative subgroups of a finite field in this fashion.
Actually, the sum-product approach also covers the structured case, although in that case it gives weaker (but still nontrivial bounds) than the Weil bound. Nevertheless, as Matt says, this is a nice demonstration of the underlying unity of mathematics, in that contributions originating from one culture can eventually impact on another.
March 22, 2013 at 9:00 pm
At the risk of self-promotion, it just occurred to me that one of my most recent papers on expanding polynomials over finite fields is basically a combination of the work of Deligne (and Weil and Grothendieck) with the work of Szemeredi, with ideas from both being absolutely crucial in order to settle the question of which polynomials over a finite field are actually expanding when applied to large subsets of that field. (At a key juncture, I need to use some etale cohomology from SGA IV in order to efficiently regularise a certain graph.) Given the recent (and very exciting) trend of algebraic geometry methods being used in modern extremal combinatorics, I can imagine that there will be several more examples of interactions between these two (ostensibly quite different) styles of mathematics in the future.
March 22, 2013 at 11:59 pm
Dear Matthew Emerton,
I am sorry, but that blog indeed requires sign-up. It wasn’t the case till the amount of spam comments turned out to be intolerable. It is rather easy to sign-up. Google recognizes a lot of ways, for example, any standard Open ID, and, of course, any Google account, which everybody using gmail automatically has. I am not particularly interested in a wide exposure (but do mind it also), and to comment the posts there is only rarely possible in Gowers’s blog (actually, such a possibility never crossed my mind).
I appreciate a lot that you had read this post. I hope that you noticed that this particular post is about me (not about T. Gowers or B. Green and T. Tao), as stated in its title. Discovering that the Green-Tao paper is not about prime numbers was indeed a big disappointment, and undermined my interest in the field quite a lot. You say that “This was something noted by several people upon the appearance of their first paper…” If it was noted only by several people, this definitely wasn’t obvious, especially to the people who just heard about it, but never attempted to study it. In fact, the Green-Tao work was widely advertised (not only by the authors) as a work about prime numbers. So, the principle of “truth in advertisement” was violated. Independently of what one thinks about this violation, the reasons for disappointment are quite clear. Any theorem about prime numbers is infinitely more interesting than a theorem about an arcane class of sets of integers. After such an experience it is hard to find motivation to study subsequent papers in the hope that some of them turn out to be about prime numbers indeed.
As I said, after this and a hardly conscious reevaluation of the “two cultures” theory, I lost interest to this activity. I wonder if there are theorems of Green, Tao, or Green-Tao which do apply only to the set of prime numbers. Juxtapositions of the sort “a theorem from number theory” + “a theorem from combinatorics” -> “a theorem about prime number” do not qualify, I am asking about a Szemeredi-like theorem which applies only to the set of prime numbers by intrinsic reasons.
I am quite happy to know that you are at least not sure that a distinction between “two cultures” exists. As it turned out, the spell of the excellent English prose in Gower’s essay eventually lost its power over me and then I was able to evaluate its content independently of its poetic appeal. There is only one mathematical culture, but, not surprisingly, different branches are at different stages of development and this leads to some difference in flavors.
Finally, I would like to point out that analytic number theory is not a “structural” branch of mathematics. According to A. Weil, analytic number theory is not a number theory at all, but a branch of applied analysis dealing with a distinct class of problems, very much like the probability theory.
The standard names of various branches of mathematics are misleading, probably, even more often than the names of theorems and basic concepts. It is well known that Pell’s equation has nothing to do with Pell. Fuchsian and Kleinian groups were discovered by Poincare. The Dirac operator was invented by Atiyah and Singer. A branch of functional analysis is called the non-commutative geometry. A branch of number theory is called the arithmetic algebraic geometry, and a couple of decades earlier was usually called Diophantine geometry. The word “geometry” is especially popular in last 5-6 decades. And a branch of analysis is called analytic number theory.
Anyhow, if we do not forget that analytic number theory is really analysis (the work of Hardy and Littlewood in analytic number theory is well know, but they were and are considered to be analysts, for example), then it hardly surprising that it can interact with another “soft” subject, the Hungarian combinatorics (quite different from another branch of “combinatorics, also called combinatorics).
March 23, 2013 at 1:24 am
To Terence Tao:
To a big extent I already replied to this comment also. I would like to add only a couple remarks. First, all this stuff is only a secondary aspect of Deligne’s work. While it is surprising and almost unbelievable that his work in algebraic geometry often provides the best available estimates of such strange at the first sight gadgets as sums of Kloosterman sums, most of people who admire his work admire it because it immensely clarifies algebraic geometry and provides key tools for moving further in algebraic geometry.
And concerning these sums of sums and other such constructs, I believe that their fate will be the same as the fate of elliptic integrals: all analysis was eliminated from the theory of elliptic integrals, and now we have a (much more general) theory of elliptic curves.
I cannot fail to notice that in all examples the applications are going in the only one (expected) direction: from algebraic geometry to combinatorics. (This reminds me of a mathematician who reportedly used to say that the games theory is important because it uses topology.) The first application (to the other combinatorics) are due to R. Stanley (and are based on the quite recent at the time results, which, if I remember correctly, also proven by Deligne). This is in complete agreement with my estimate of the relative depth of these branches of mathematics. (Probably, in Stanley’s combinatorcs there are some minor applications in the other direction.)
March 23, 2013 at 1:57 am
Dear Sowa,
Weil claimed that analytic number theory is not number theory, and that Hardy was not a number theorist; that doesn’t make his claim true. One could note that Deligne himself used analytic number theory methods in some arguments (among other possible reasons, as a way of observing that analytic number theory has something to contribute outside itself). One could also note that Hardy was the first to prove that there were infinitely many zeroes on the critical line, and that his argument used the description of the zeta function as a Mellin transform of an automorphic form, and the properties of this automorphic form — that looks like number theory (indeed, one of the deepest parts of number theory, namely the relationships between zeta functions and automorphic forms) to me.
(And, as an aside about the role of analytic number theory in some of the other topics under discussion, one should say that there is no doubt that the impetus for the Riemann hypothesis in Weil’s conjectures came from the original Riemann hypothesis in analytic number theory.)
As I pointed out, there have been applications of additive combinatorics to number theory: the work of Green–Tao on linear equations in primes (with precise asymptotics, and building on Vinogradov’s method among other things, hence very much about primes as far as I can tell), and the sieve of Bourgain–Gamburd–Sarnak.
In conclusion, I think that the various mathematical topics under discussion here are more unified than is suggested by your description of the situation. But perhaps we will just have to disagree.
Regards,
Matthew
March 23, 2013 at 5:34 am
Dear Matthew Emerton,
The classification of Hardy as an analyst has nothing to do with A. Weil at all, and I am not aware of any attempt to contest this idea. His work on zeroes of the zeta-function is a work of an analyst. Littlewood wrote the problem of zeroes was a popular problem among the analysts. Littlewood knew about the problem very early, but had no idea about any connection with the prime numbers. For him it was just an intriguing problem in the theory of holomorphic and meromorphic functions. Of course, later on he learned about the connection with primes. Vinogradov’s work is a version of the method of Hardy-Littlewood. He authored the worst textbook in number theory I ever looked at; it is quite clear that he had no taste for any sort of number theory.
A. Weil indeed wrote that analytic number theory is not number theory. But he also wrote an explanation of what he means by this. It is not very important how do we call some branch of mathematics (or Pell’s equation), but some understanding of the nature of various branches is important. Let as call the analytic number theory in exactly this way, and let us call Weil’s number theory the branch of mathematics he had in mind. Since he wrote a couple of books about this branch, another book about its history, it is not hard to sort out what is Weil’s number theory. The point of Weil’s remark is that these two areas, the analytic number theory, and the Weil’s number theory are quite different in many respects, and that it would be better if we openly admit their difference. It seems to me that this is indeed true.
As of your conclusion, I am surprised that you are suggesting to agree to disagree. It seems to me that this is the issue where our positions are very close. The idea of the unity of mathematics is rather dear to me. The continuum connecting Weil’s number theory with analytic number theory is a strong argument for this unity. I see here only one possibility for some disagreement: I don’t think that the unity of mathematics implies that all its branches are equally deep, equally well developed, etc. To hold the latter position is like to believe that all problems are equally difficult. But I did not notice that you claimed anything like such equality. So, at least right now there is no disagreement about the unity.
sowa
March 23, 2013 at 10:25 am
From sowa’s blog:
I think that the implicit assertion here — which one might caricature as the assertion that as branches of mathematics mature they become more and more like modern algebraic geometry — is a very interesting one, because it is neither obviously true nor obviously false. As I tried to argue in “The two cultures of mathematics” it does not appear to be true in extremal combinatorics, unless one generalizes the notion of “conceptual framework” to allow guiding principles that cannot necessarily be encapsulated in the form of concise definitions and lemmas. There is also an intermediate possibility, which is that it is possible to create a conceptual framework of that kind for combinatorics, but only in a somewhat artificial way that is less convenient to use than less precise guiding principles.
However, I have no proof that the kind of organization that sowa likes will never happen in a satisfactory way in extremal combinatorics. If I had been alive in the 19th century, it would never have occurred to me that continuity could be encapsulated so neatly in terms of open sets, and maybe we are all failing to spot some similar future development for extremal combinatorics.
March 23, 2013 at 10:30 am
As an afterthought, it occurs to me that the recent development in combinatorics that proves finitary results such as Szemerédi’s regularity lemma using infinitary methods that get rid of a lot of complicated dependences between small real parameters could be regarded as making parts of extremal combinatorics “less elementary, but more clear”. Terence Tao discusses this kind of approach in many places in his blog.
March 23, 2013 at 3:35 pm
I have a feeling that sowa does not really divide mathematics into ‘structured’ and ‘unstructured’, or ‘conceptual’ and ‘non-conceptual’, but into mathematics that requires ‘soft’ arguments and ‘hard’ arguments. Either one never did ‘hard’ mathematics or got tired of doing ‘hard’ mathematics, having sufficient success with ‘soft’ mathematics, and now rationalizes it by looking down on anybody doing ‘hard’ mathematics.
The fact is that there are many problems in mathematics that by their nature require ‘hard’ arguments. Imagine, for example, studying the asymptotics of a solution of some complicated PDE. There is no amount of conceptualizing that will make it ‘soft’. Probably, even the definitions that are needed to formulate the solution might be too ‘hard’ for a taste of a ‘soft’ mathematician.
Paul Erdős was fond of proofs from ‘The Book’ and nobody can doubt that he had the depth to appreciate the beauty of mathematics. It is also true that there are many proofs from ‘The Book’ that involve ‘hard’ arguments, discovered by mathematicians whose depth will match that of any ‘soft’ mathematician.
March 23, 2013 at 3:55 pm
That’s an interesting way of thinking about it. I think I’d want to add a small qualification, which is that some of the mathematics that sowa likes involves hard arguments, but it packages them up so that when you have done the hard stuff once, you can use it as a black box in a softer way later. An example that springs to mind is proving Bott periodicity: you have to do some work, but once the result is established, you don’t have to repeat that work and can apply the result in many places. It is of course wonderful when that kind of packaging is possible.
March 23, 2013 at 4:25 pm
The example of elliptic integrals and elliptic curves, to me, is actually quite a good example of what structured mathematics is, and what its boundaries are. We have many examples of the beautiful crystalline structure of the mathematics surrounding elliptic curves being replaced by the quite different (but to my mind, still very beautiful) disorder and anti-structure when one tries to move to more general mathematical settings. For instance, when solving completely integrable equations such as the periodic Korteweg-de Vries equation, there are a beautiful set of special solutions (coming from finite gap potentials) in which the equation can be solved exactly using elliptic integrals (or more precisely, Abelian integrals which are a hyperelliptic variant), giving quasiperiodic solutions. However, when one moves to arbitrary data (with infinitely many gaps in the spectrum of the associated Lax operator), then the situation is now a nonlinear version of a Fourier series with arbitrary coefficients, and is as chaotic and disordered as one would expect from Fourier analysis. Nevertheless, we can still extract finite gap approximants to such infinite gap solutions, thus seeing the core structured behavior amidst the general chaos. In nonperiodic settings the analogue would be what is (conjecturally, at least) a “resolution of solitons” phenomenon in which the structured component and the radiative component of the solutions can be separated asymptotically in the limit, which is a sort of nonlinear version of the way that the spectral theorem can distinguish discrete spectrum from continuous spectrum. Again, the radiative component is not structured at all, being again like an arbitrary Fourier integral, and it complements the structure of the soliton component rather than being somehow “inferior” or “softer” than it. The interplay between structured components of a PDE (e.g. ground state solitons) and radiative or perturbative components is an incredibly rich subject, requiring a combination of both structured mathematics and unstructured analysis; to loosely quote Tolstoy, “true life is lived when tiny changes occur”.
Or: the rational points on an elliptic curve have an enormous amount of deep structure, of course, starting with the basic fact that they form a finite rank abelian group. But when one moves to higher genus what one sees instead is antistructure: the structure of an integer point in a higher genus curve has the tendency to destroy or repel most other possible integer points, which is what ultimately leads to results such as Faltings’ theorem (Mordell conjecture) on such curves. This type of result is much closer in spirit to what comes out of what Grothendieck called the “arid steppes” of analysis (such as Fourier analysis): analysis tells us that when analysing the Fourier coefficients of an arbitrary function, then it is occasionally possible to have a single large Fourier coefficient (which, in this context, is the structure playing the role of an integer point on a high genus curve), but this type of structure tends to repel other such structures, so that a given function cannot have too many large Fourier coefficients at once. To encapsulate this fundamental antistructure phenomenon one needs tools from analysis (in this case, things like the Plancherel identity or Bessel’s inequality) than from the structured side of mathematics. And we know from various “no go” theorems (e.g. Matiyasevich’s theorem, unsolvability of the quintic by radicals, etc.) that once one’s varieties reach a certain level of complexity, it is no longer reasonable to expect the same paradise of structure that elliptic curves enjoy in abundance. This is not just a reflection of the relative state of development of the genus one and higher genus theory, or of the relative “depth” or “importance” of each one; it is a fundamental distinction arising from the nature of mathematics itself.
The story of Szemeredi’s theorem and its variants can be viewed as a struggle to usefully separate out the structured and unstructured components of a mathematical object, in this case a set of integers and the additive patterns it contains. The way my first result with Ben worked, we were able to use tools from combinatorics and analysis to show that the primes could essentially have only a bounded number of structures in them that could influence the number of arithmetic progressions it contained, because each such structure tended to repel all the others. And then we used Szemeredi’s theorem to conclude that no matter where these bounded number of structures were distributed, arithmetic progressions would necessarily be generated. As correctly noted above, this is a result more about progressions than about primes (my coauthor, Ben Green, tends to stress this point in his own expositions, e.g. in Section 2 of http://arxiv.org/pdf/math/0508063v1.pdf ).
In my later work with Ben (and also Tamar Ziegler) on generalising this result to further additive patterns and to obtain precise asymptotics on the counts for these patterns, the primes play a more essential role. Firstly, one has to use deeper tools from analysis (in this case, the inverse conjectures for the Gowers norms) to make the “bounded number of structures” mentioned earlier far more algebraic, and in particular to give them the structure of a nilsystem. Then, in order to keep the primes additively disjoint from such nilsystems, one now has to exploit the antistructure effect encoded by the multiplicative structure of the primes, which tends to repel additive structure (this is yet another variant of the sum-product phenomenon mentioned in previous comments). Roughly speaking, when the nilsystem is coming from an “irrational” shift, then one can use the Vinogradov-Vaughan method of bilinear sums to formalise this repulsion between multiplicative and additive structure. In the case of rational shifts, one instead has to use the classical device of L-functions, and ultimately turn to Siegel’s theorem on the zeroes of such L-functions, which again ultimately boils down to an antistructure phenomenon in which one L-function zero close to 1 tends to repel all other such zeroes. At the end of the day, it is true that our arguments do not discover any new structure on the primes beyond the multiplicative structure that was already known to be present – but then again, all our conjectures about the primes (e.g. the Mobius randomness conjecture) point to the fact that no further such structure should be expected (at least within the additive framework considered here), and instead it is the antistructure of the primes which we have been able to shed some new light on rather than the structure of the primes. Again, this antistructure is an actual feature of the primes (particularly when they are viewed additively) and is not likely to be supplanted by a structured viewpoint in any time in the near future. (Even in the function field setting, for instance, where there is substantially more structure as evidenced for instance by the Weil conjectures, the primes (i.e. irreducible polynomials) are still extremely anti-structured, with only a very small minority of primes in this case being able to be manipulated explicitly (though this situation is much better than in the rational case in which we cannot really get our hands on a single large prime at all). This small set of structured primes in the function field world is enough to solve some qualitative problems that the rational case cannot (e.g. the twin prime conjecture is known in function fields), but for quantitative conjectures such as the asymptotics for twin primes, the situation is essentially just as difficult as in the rational case.)
March 23, 2013 at 5:07 pm
To summarise: the more algebraic areas of mathematics are generally in the business of establishing ever-stronger lower bounds on the amount of structure present in some mathematical object of interest, whereas the more analytic areas of mathematics are generally in the business of establishing ever-stronger upper bounds on such structure, and nowadays there are now many areas of mathematics in which there is a crucial interplay between the two sides. But it would be a mistake to judge the stature of one of these sides of mathematics through the lens of the other, either by dismissing analysis through its lack of new structures discovered, or dismissing algebra through the lack of new upper bounds discovered.
March 25, 2013 at 2:19 am
To T. Gowers:
There is a lot of quite interesting comments now, and some of them call for an extensive reply rather unsuitable for a comment, especially a second level one. In particular, my reply to your comment above is fairly long. So, I posted it as Reply to Timothy Gowers.
If you will be inclined to comment there, you can use your WordPress account for authorization (I was forced to introduce authorizations by spam). This would amount to, I believe, just selecting WordPress from a menu and pressing a button. Even if your reply will be posted here, a reference there would be very much appreciated: I do not get any e-mails from this site except invitations to join WordPress, but I get all notifications from Google.
March 26, 2013 at 2:27 am
To T. Gowers:
I was planning also to comment on your remarks about hard and soft mathematics and about Bott periodicity. My comment are again quite long and posted as Hard, soft, and Bott periodicity – Reply to T. Gowers.
March 26, 2013 at 7:47 am
>I cannot fail to notice that in all examples the applications are >going in the only one (expected) direction: from algebraic >geometry to combinatorics.
arXiv:1209.0207
Rational points on pencils of conics and quadrics with many degenerate fibres
Tim Browning, Lilian Matthiesen, Alexei Skorobogatov
(Submitted on 2 Sep 2012)
For any pencil of conics or higher-dimensional quadrics over the rationals, with all degenerate fibres defined over the rationals, we show that the Brauer-Manin obstruction controls weak approximation. The proof is based on the Hasse principle and weak approximation for some special intersections of quadrics, which is a consequence of recent advances in additive combinatorics.
——————
arXiv:1202.1185
Some Applications of the Hales-Jewett Theorem to Field Arithmetic
Authors: Bo-Hae Im, Michael Larsen
Categories: math.NT Number Theory (math.CO Combinatorics)
Comments: 10 pages
MSC: 05D10, 11G05, 12E30
Abstract: Let $K$ be a field whose absolute Galois group is finitely generated. If $K$ neither finite nor of characteristic 2, then every hyperelliptic curve over $K$ with all of its Weierstrass points defined over $K$ has infinitely many $K$-points. If, in addition, $K$ is not locally finite, then every elliptic curve over $K$ with all of its 2-torsion rational has infinite rank over $K$. These and similar results are deduced from the Hales-Jewett theorem.
March 26, 2013 at 1:48 pm
To Emmanuel Kowalski:
This looks very convincing for some, probably. But before your comment all tried use really significant and already known to relatively wide audience results. Now you pick up two highly specialized short notes which mention that some combinatorial theorems are used. The abstracts look like their authors (most of whom are known to me as mathematicians) met at some conference and proved a couple of liitle results during a break.
What a surprise! I wrote about this exactly in the post linked there. Combinatorial arguments are used everywhere. This is one of my main reasons not to believe in the existence of “two cultures”.
You need to try harder. It is not a big deal to find in arXiv a paper with some desired properties.
March 26, 2013 at 5:57 pm
I don’t particularly believe in two cultures either (except maybe open-mindedness versus closed-mindedness, which seems to me to be a psychological reality), but since there was a claim of applications going in one direction, why shouldn’t examples in the opposite direction be relevant?
(Note: I have no idea what “before your comment all tried use really significant and already known to relatively wide audience results” could possibly mean).
————————————————–
arXiv:1103.3423
Title: Generalizations of the Kolmogorov-Barzdin embedding estimates
Authors: Misha Gromov, Larry Guth
Categories: math.GT Geometric Topology
Comments: 45 pages
MSC: 53C23
Abstract: We consider several ways to measure the `geometric complexity’ of an embedding from a simplicial complex into Euclidean space. One of these is a version of `thickness’, based on a paper of Kolmogorov and Barzdin. We prove inequalities relating the thickness and the number of simplices in the simplicial complex, generalizing an estimate that Kolmogorov and Barzdin proved for graphs. We also consider the distortion of knots. We give an alternate proof of a theorem of Pardon that there are isotopy classes of knots requiring arbitrarily large distortion. This proof is based on the expander-like properties of arithmetic hyperbolic manifolds.
—————————————————
GAFA, Vol. 12 (2002) 330 – 354
COUNTEREXAMPLES TO THE BAUM–CONNES
CONJECTURE
N. Higson, V. Lafforgue and G. Skandalis
Quote (there is no abstract):
“Guoliang Yu [Y] proved that the coarse Baum–Connes conjecture is true for any bounded geometry metric space which admits a uniform embedding into Hilbert space. At the time Yu announced his result there were no known examples of bounded geometry spaces which did not admit such an embedding, but Gromov pointed out that no expanding sequence of graphs so embeds. And indeed it turns out to be rather simple to construct a counterexample to the coarse Baum–Connes conjecture starting from a suitable expanding sequence.”
——————————-
It must be nice to prove such results after meeting quickly at a conference!
March 26, 2013 at 7:46 pm
For what it’s worth, at least the way I have had it presented to me Hironaka’s theorem on the resolution of singularities is based on reducing the the problem from algebraic geometry and then solving a problem relating to an elementary combinatorial game.
http://www.ams.org/journals/bull/2003-40-03/S0273-0979-03-00982-0/home.html
March 26, 2013 at 8:06 pm
Dear Emmanuel,
I think “before your comment all tried use really significant and already known to relatively wide audience results” means “before you made your comment, every one [making the argument that there are applications in the other direction, i.e. from the second culture to the first culture] tried using as examples results that are
really significant and known to a relatively wide audience”. I don’t know any Russian grammar, unfortunately, but this reminds me of constructions in German where what would be a separate phrase in English becomes instead an extended adjective that is directly applied to the noun.
Regards,
Matthew
March 27, 2013 at 12:36 am
Wow!
To Matthew Emerton:
That is a fantastic insight! You are almost right on the target. This elevates the discussion to a new level rarely seen on the web.
But the lack of knowledge of Russian grammar apparently misleads you a little. I happen to know the Russian grammar a little and somewhat better the German one. When I write in any language, I indeed tend to write in way which is more natural for the German language. When I write in English, I often have to go over the text and to break complicated German-like phrases into several more suitable for English. This writing style is more or less grammatically correct in Russian (except some difficult German constructions having no equivalents in both Russian and English). But it is fairly alien to the Russian language. Apparently, if I would write the same things in Russian, the result will look as a translation from German.
It seems that when I write in English, it also looks like a translation from German.
This has nothing to do with my language skills or lack of them. When I write in English, I think in English. What you see here is not a translation from any other language. (I just split a phrase into two!).
The reason is quite relevant for mathematics. I do not think linearly no matter what I am thinking about, a theorem, a TV show, or what I would like to do during the Spring break. Some people claim that they think in words and hence almost inevitably they think linearly. Perhaps, this style, if it really exists, is suitable for working in the mathematical logic.
But mathematics is not a linear subject. A more adequate model would be not a sequence of statements, but a graph which is even not a tree (combinatorics!). Still, oriented cycles are not allowed. 🙂 My own mathematical results could not be discovered by linear thinking alone, I believe.
The German manner of writing allows communicating at least a part of this nonlinearity (for example, tree-like fragments). Attempting to communicate my ideas adequately, I am lead to German-like constructions very often. Obviously, this is not working fairly often; may be this is newer works.
March 27, 2013 at 1:00 am
To Emmanuel Kowalski:
It seems that you inclined to communicating by citations alone. In my opinion, using the abstracts alone is highly misleading.
This time the abstracts do not mention any recent results. Apparently you indeed tried harder and cited papers which I am familiar with (perhaps, you even managed to find out what is my real life identity) and which are widely known. There is a lot to be said about them. For example, your second citation is completely irrelevant for the current discussion. The result depends only on the existence of expander graphs proved long ago by G. Margulis by highly conceptual methods (if I remember correctly).
A proper reply to your citations, apparently supposed to prove something, would be a detailed review of every cited paper, geared toward not the main results but to analysis of what combinatorics is involved and how.
This does not seem to be an appropriate exercise in the comments. Such things are usually done only in monographs about the history of mathematics. Last year event can already be history, but I am not inclined to start writing such a book, especially to start in comments.
March 27, 2013 at 1:32 pm
“This time the abstracts do not mention any recent results”
If 2011 is not recent, of course.
I preferred communicating by citations because almost every other sentence I started writing seemed to involve explicit or implicit assumptions concerning Sowa. But I have no way of checking whether these assumptions are correct or not…
I am making no claims of any kind concerning combinatorics (I am very bad at combinatorics myself, almost as bad as with general topology). I’ll just make one point related to another remark of Sowa somewhere in the discussion: I certainly believe that definitions can, indeed, be crucial means of making progress and increase understanding in mathematics. And expander graphs are, to me, one of the perfect examples.
March 27, 2013 at 4:06 pm
sowa : A tiny (but, I think, very relevant) correction. The existence of expander graphs was known long before Margulis’s work. Indeed, the probabilistic method of Erdos gives a very short nonconstructive proof! See section 9.2 of Alon and Spencer’s book “The Probabilistic Method”. What Margulis did is show that certain families of Cayley graphs are expanders, which gave the first explicit construction of them. Margulis’s proof is beautiful and instructive, but if all you want is existence then the combinatorics proof is much more elegant and conceptual.
March 27, 2013 at 7:45 pm
Dear Sowa,
I only intended to explain the meaning of the sentence to Emmanuel, who seemed genuinely confused. I now also know a little more about Russian grammar (or least, have had a momentary misconception clarified), and a little more about your writing/thinking style.
Regards,
Matthew
March 28, 2013 at 1:03 am
To Emmanuel Kowalski:
I meant the “combinatorial” results used, not the year of the paper. I thought this is obvious from the context. Sorry.
I put above the quotation marks because it is really hard to consider anything done by Kolmogorov as combinatorics.
The rest is posted in The value of insights and the identity of the author. I would like to entice you to follow the link. There is an offer for you there.
Sowa
March 28, 2013 at 1:25 am
To Andy Putman:
I did not intend to say that the method of Margulis is the only one. Moreover, I said somewhere here that I believe that the proper context for the theory of expander graphs is analysis and combinatorics.
Still, the existence of expander graphs can be proved by methods of Margulis and this makes the cited paper independent of combinatorics. Second, an explicit construction is usually preferred to a pure existence results.
Finally, I agree that probabilistic methods in combinatorics are powerful, and sometimes elegant in their special way. But I do not agree that they are conceptual. The reason is that they do not clarify the results proved by them. You calculate or just estimate some probabilities (in finite situations this is equivalent to counting), find that for probabilities A and B of two different properties, verify that A+B>1, and you know that an object having both properties exists. But you have no idea why these properties are compatible.
So, I do not feel that the probabilistic proof of the existence of expander graphs is interesting. From my point of view (I definitely said this already) the expander graphs are interesting only because Margulis-like methods can be used to prove their existence, and they can be used to prove result like the results of the cited paper.
March 22, 2013 at 12:42 am |
Well anyway, I liked this write-up a lot; the talk must have been great. I especially liked the quote from Katz on independence of
, in the section on the final Weil conjecture.
March 22, 2013 at 1:26 am |
Oops, that wasn’t meant to be anonymous. Forgot to log in.
March 22, 2013 at 6:09 am |
@Sowa,
That would be a long winded discussion. But all that aside, could you link to the S. Maclane article? I would like to read it but haven’t been able to find it.
March 22, 2013 at 10:41 am
To Shubhendu Trivedi:
It looks like that one needs to make a trip to a physical library in order to read this note. I failed to find an electronic version. Here is the MathSciNet entry for this note, entirely. The note is 2 pages long, the review – 2 lines.
MR0885540 (88f:00019)
Mac Lane, Saunders(1-CHI)
Criteria for excellence in mathematics.
Bull. Soc. Math. Belg. Sér. A 38 (1986), 301–302 (1987).
00A25
The author lists the following qualities of mathematical research and discusses them: inevitability, illuminating, depth, relevance, responsiveness, timeliness.
March 22, 2013 at 8:31 am |
Is sowa Sheldon Cooper?
March 22, 2013 at 2:37 pm
No, his name is Professor Pnin.
March 22, 2013 at 2:56 pm
There should be a TV series based on the character of Professor Sowa – a prominent French-Russian mathematician who hates Hungarian-style combinatorics, but loves Hungarian style cheesecake. He hates Endre Szemerédi, and he adores Zsa Zsa Gabor. Because of this, his state of mind is always off balance and, as a result, all his results are proved by contradiction.
March 23, 2013 at 12:53 am
I shouldn’t be interested in this but curiosity got the better of me.
That is interesting. I did notice that Sowa has a livejournal that he writes in Russian.
March 22, 2013 at 7:21 pm |
[…] us with an easy to read essay about the Abel Prizewinner’s research for nonexperts. You can find a link to it here on his blog. We do have to quote his opening paragraph, […]
March 23, 2013 at 2:48 am |
I just hope that Sowa is not the infamous Lubos. That fills the physics blogs with hot air.
March 23, 2013 at 1:21 pm |
No, he is not Lubos. He is a good mathematician, not an A-star like Gowers, but certainly an accomplished mathematician, who was not gettings any grants lately. And so he was approached by a certain publisher and was given a very specific task. All this is good old Elsevier money
March 23, 2013 at 1:35 pm
The suggestion about Elsevier is complete nonsense. Sowa is 100% on the side of Timothy Gowers on this issue.
March 24, 2013 at 12:56 am |
sowa writes: “Discovering that the Green-Tao paper is not about prime numbers was indeed a big disappointment, and undermined my interest in the field quite a lot. You say that “This was something noted by several people upon the appearance of their first paper…” If it was noted only by several people, this definitely wasn’t obvious, especially to the people who just heard about it, but never attempted to study it. In fact, the Green-Tao work was widely advertised (not only by the authors) as a work about prime numbers.”
My experience was exactly the opposite; from the very beginning, I thought it was presented very clearly (perhaps more so by the authors than by others) that the work of Green and Tao was not primarily about prime numbers, but about general structure theorems about subsets of the integers. And for me this was not a disappointment at all, because — is it bad to say this? — I actually DO NOT CARE whether or not there are long arithmetic sequences in the primes.
But I DO care about general structure theorems on sets that give criteria guaranteeing the existence of long APs (and now, with the aid of Ziegler and others, long polynomial progressions…) and I care about these even more given that they’re now understood to be they’re embedded in a suite of results involving sums and progressions in more general groups.
Of course, my experience is no more valid than yours! But I just wanted you to know that yours was not universal, even among firmly “first culture” mathematicians like you and me.
March 24, 2013 at 4:18 pm |
To a large extent, this post should have been a celebration of Pierre Deligne and algebraic geometry, so I hope it’s not too late to ask this question? This Abel Prize put a spotlight on some of the earlier fundamental results in the field and put them into historical perspective beautifully. To complement this, I would be very interested to hear about big trends and fundamental advances in this area in more recent times? I understand that this is subjective, but there must be five top things that most experts would put on their list.
March 24, 2013 at 4:36 pm |
Another interesting issue raised on sowa’s blog was the unfairness (stupidity?) of the Fields Medal eligibility rule. Just for fun, let me propose a rule that would smooth out the cliff effect of the current rule:
(a) the results for which the medal is awarded should be either published or made publicly available (e.g. on arxiv) when the author is 38 years old or younger;
(b) the person should be 42 years old or younger on August 1st of the year of the award.
The first part of this rule is already in effect, essentially, so there is no drastic historical discontinuity. The second part creates a buffer to make the cliff effect more fair. This rule is not perfect, but it would make borderline cases much less likely.
March 24, 2013 at 6:52 pm |
Test comment.
March 24, 2013 at 6:55 pm |
Hey Tim, Just a quick test for you to see if it goes to the moderation queue …
March 24, 2013 at 7:42 pm |
This is a test comment
March 24, 2013 at 8:14 pm |
This is a test comment to another post as requested.
March 24, 2013 at 9:33 pm |
I enjoyed the text immensely. Thank you! At the same time this serves as a test comment.
March 24, 2013 at 11:14 pm |
Hi.
March 25, 2013 at 1:02 am |
Test comment
March 25, 2013 at 1:58 am |
Test comment Re: moderation queue.
March 25, 2013 at 3:34 am |
To JSE:
My reply to you also turned to be relative long. I posted it as Reply to JSE. Your WordPress account is sufficient to write comments there, if you like.
March 25, 2013 at 3:38 am |
It looks like comments are moderated now. My comment awaiting moderation acquired a strange link in the process of posting, so I would very much appreciate if it will be deleted. I will try to post the correct comment.
March 25, 2013 at 3:39 am |
To JSE:
My reply to you also turned to be relative long. I posted it as Reply to JSE.
Your WordPress account is sufficient to write comments there, if you like.
March 25, 2013 at 2:18 pm |
[…] 一个新近的例证是P.Deligne获得了今年的Abel奖。在T.Gowers宣布这一消息的博客文章里，一位化名sowa的数学家公开质疑 […]
March 25, 2013 at 2:21 pm |
Sowa says in his response to Tim Gowers: “… it seems that there are only the following options for a mathematical theory or a branch of mathematics: to continuously develop proper conceptualizations or to die and have its results relegated to the books for gifted students…”
Sowa, this is wishful thinking that conveniently fits your narrative. Mathematical theories ‘die’ not because they are not conceptual enough, but because they are unmotivated and find no applications. Hungarian-style combinatorics has many practical applications (already mentioned above, e.g. in computer science), so it’s unlikely to ‘die’ in the sense that you described. Every useful and well-motivated field will survive, and it will naturally mature as people find simplifications, clarify concepts, establish connections, etc.
I recently read a quote (a bet) by Voevodsky that mathematics as a science will not exists in 30 years. Is there something going on that makes algebraic geometers so pessimistic? Is modern algebraic geometry being suffocated by abstraction and conceptualizing?
March 25, 2013 at 2:37 pm
Do you have a source for that bet? I’d be interested to look up the context.
March 25, 2013 at 3:00 pm
Correction: 50 years. It is a part of this interview (in Russian): http://www.polit.ru/article/2006/08/22/voevod/
A relevant paragraph can be translated roughly as follows:
“Even if there is no nuclear war in the near future, I do not expect anything good happening to mathematics. Mathematics have been developing intensively over a long period of time, there were many big breakthroughs. But mathematics that we have today… we are spending unnecessarily large resources: time, human resources, financial resources. You see, in modern science the situation is such that the time a person must spend just to understand a problem is unacceptably high. I can not explain even to a very good graduate student all the details of my work! Today it is harder and harder for new people to engage in the scientific process. I think this is a bad sign. If mathematics does not make a turn toward practical needs of the mankind, in fifty years it will not exist in its present form.”
His friend and colleague Yuri Shabat objects and they make a bet, to be evaluated in 30 years, since they both don’t expect to be alive in 50 years.
March 26, 2013 at 12:26 am |
To T. Gowers:
The quoted passage by Volodya Voevodsky should not be taken out of its context. For example, it is immediately preceded by the following: “…it is already obvious that thermonuclear weapons, which earlier were hardly accessible, soon will be easily available. I don’t see any reasons which may stop people having a desire to use them. Obviously, a nuclear war awaits us within few decades. Although I must say that according to the American scientific journals, like “Science”, which I am following, claim that the aftermath of a nuclear war is not as scary as we may expect.”
If one knows Volodya personally at least a little, these and similar statements by him would tell much more about his mood at the moment than about anything else.
There is also a much broader context for statement about “practical needs of mankind” and other similar issue. The top class mathematicians who grow up in Soviet Union made such statements, and, moreover, changed their areas of research in accordance with such statements, incomparably more often than their Western colleagues. Pontrjagin did this (when Serre in his thesis dramatically solved several problems, which occupied Pontrjagin for about 15 years); Novikov did this (I will refrain from telling what was the reason according to some of his students). Others simply worked on military problems for a period of time and then returned to the pure mathematics – this was the most secure way to advance your career, and often the only way. While right after the WWII many managed to find some moral justification for designing atomic and later nuclear weapons for Stalin (or replicating Western designs stolen by spies), by 1970 this was impossible, so the mathematicians moving in applied areas started to invent reasons more acceptable to the public and to their colleagues. Anyhow, there is a deeply ingrained among the soviet scientists tradition of moving to something more or less applied when they start to feel that they are already past their prime. It would be hard to explain why phenomenon this phenomenon survived till now, and continues to exists even among people who left USSR 40 years ago.
In contrast, the Western society created a lot of obstructions for changing areas in any direction.
March 27, 2013 at 7:10 am |
[…] and this blog as well. (A few more links: my most decorated MO answer is about Erdős, a recent heated discussion on the “two coltures in mathematics,” a new post on Erdős discrepancy problem on GLL, […]
March 27, 2013 at 9:54 pm |
A few remarks:
1) I like exploring the Internet as a tool for academic discussion and research and therefore I was a little worried when this discussion started. But overall, remarkably, the discussion moved to the more interesting issues and it is quite interesting and educating.
2) Personally, I am a great fan of combinatorics which is my area. My approach about it is similar to my approach toward other choices I made or choices made for me. To be a combinatorialist is a good choice for one life time. Also to be a mathematician, to be in academia, to live in Israel, all are good choices for one life time, which is incidentally mine. (There is a single exception to this approach regarding my wife and family that I would take with me again and again, to any life time.) Because of this, I never cared too much about the status of combinatorics. I did not mind too much when early in my career combinatorics was not highly considered, and when in the 90s Isreal Gelfand visited Jerusalem and made a statement that combinatorics is the most important area of future-mathematics, I did not mind it too much as well.
3) I am quite interested in people’s views about mathematics, and this discussion give a nice opportunity to learn about various points of view, including sowa’s. (This is also why I like papers like Gowers’s “two cultures” paper.) It is quite fascinating how people can have different interpretations of reality. The point of view of ‘M’ that sowa mentioned is exciting as well, and we all know who he is, don’t we? (The head of MI6 among other things.)
4) Regardless of the status of combinatorics, Endre Szemeredi is such a very rare genius in the landscape of mathematics and mathematicians of our time, that sowa’s assessment regarding Endre, is, in my opinion, vastly unreasonable.
5) Regarding the presentation of the Abel prize winner: Tim Gowers is not a good speaker. Tim is a superb speaker. Maybe it will be enough to settle for an excellent but not quite a superb speaker but there aren’t so many of this kind either. Tim is also a Field medalist. Again, maybe it will be enough to settle for a larger circle of world-class mathematicians, but this is also a rather exclusive set. And third, Tim is willing. And now, this may come as a surprise, to you, sowa, but it is not so easy to find people who are willing to present the achievements of other mathematicians (and to devote much of their time for the task). The identity of the mathematician presenting the freshly chosen Abel laureate is not terribly important, but it is quite important for the prestige of the Abel prize and for promoting the cause of mathematics through the prize. Having Tim as the presenter is a remarkable success for the Abel prize officers.
March 30, 2013 at 3:16 am
Professor Kalai,
Thanks for sharing that bit about Gelfand and combinatorics. I had often looked for something concrete on the internet as I had often heard that he was sympathetic to combinatorics from his disciples. I had often only found this extremely insightful article by Doron Zeilberger that mentions it in passing somewhere in between: http://www.math.rutgers.edu/~zeilberg/Opinion62.html
Recently somebody pointed this article to me (incidentally also by Zeilberger) http://www.math.rutgers.edu/~zeilberg/Opinion1.html although I barely qualify as a novice, this immediately made an impact (immediately putting Tim Gowerss essay in better perspective for me personally). Especially because I had studied some of those ideas in a Graph Theory textbook by Bela Bollobas (Modern Graph Theory, the last chapter).
March 31, 2013 at 7:09 am
То Shubhendu Trivedi:
If you are inclined to take statements of Doron Zeilberger seriously, on the face value, then it is hard to understand why you would like to be a mathematician. Perhaps, you a familiar only with the cited ones. Then it would be worthwhile to read other “Opinions”, as also some publications.
His view of the future of mathematics and mathematicians is rather bleak: theorems are proved by computers (he already published some results proved by his computer), and mathematicians are no more than technical assistants to these computers. I would prefer to be at least a software engineer in such a case, or do something completely unrelated to such strange activity.
By the way, T. Gowers holds a similar position. It was discussed a year ago in his blog on the occasion (surprise, surprise) of 2012 Abel prize.
I think that the fact that at least two leading experts in combinatorics predict such a future is not an accident. Perhaps, the combinatorics indeed can be done by computers with minimal human assistance.
Concerning specifically Opinion 62 of Zeilberger, I should say he made so many factual mistakes in so little space, that it is really hard to take his conclusions seriously.
March 31, 2013 at 9:22 pm
I’m not suggesting anything of that sort. But there was enough in it (op 1) to make me think about the issue. (about 62, sure may be. But I linked to it only because of the mention of combinatorics and Gelfand. I thought I was pretty clear about that).
April 1, 2013 at 4:08 am
To Shubhendu Trivedi:
It seems that I have to provide the details. See
D. Zeilberger’s Opinions 1 and 62.
April 1, 2013 at 6:39 am
Sowa,
This is a zero information comment. But thanks for writing up a detailed comment. I will read your article with interest.
March 28, 2013 at 3:01 am |
To Gil Kalai:
1. It seems that mathematicians usually hardly realize what opportunities the internet provides. For example, all problems with the big publishers can be solved by internet alone, without any new journals, boycotts of Elsevier, etc.
Of course, I was very happy when the discussion ceased to be focused on my personality and turned to more interesting matters.
I am still planning to write a reply to Terence Tao, but when I finished reading his recent comments it was already clear that this would not be an easy task (they are too technical for a quick reply). It may happen that a reasonably full and clear reply requires writing a book. Then I will have to limit myself by few remarks.
2. I would be very surprised if this wouldn’t be your position.
3. I still do like the “Two cultures” essay. But the initial interest (may be this is all correct, this would be tremendously interesting!) is gone and I am firmly convinced that this theory is wrong.
4. I wish somebody would convince me that your opinion is correct. But even T. Gowers failed here. Any reference, preferably not very demanding for an admirer of Serre, Grothendieck, Atiyah, Quillen, etc. would be greatly appreciated. The problem results from the fact that by now I know a lot about both purported cultures, and can compare their results. This was not the case in 2000, say.
5. Well, your position is inevitably leading to a question I had already asked in a weaker form: is T. Gowers going to be the presenter of winners for life? All your arguments apply to the next year, to the year after next, and so on.
No, this is not a surprise. Fairly long ago I reached the conclusion that the exposition of other mathematicians’ works is really valued only in France. I am immensely grateful to French mathematicians for all their seminars devoted to the result of other mathematicians, and especially to the Séminaire N. Bourbaki. Apparently, this was valued to some extent in the now defunct USSR, but hardly at a level comparable to France. And this is not valued at all in the US (and I believe that this is neither an accident nor a temporary phenomenon).
Unfortunately, I am not a Fields medalist (no grudges here). 🙂 I was told more than once that I am a good speaker and this very well may be the case, but I will not believe to anybody who will say that I am a superb speaker. I was always interested in works of other mathematicians and was giving talks about other mathematicians results till I found myself in a situation when nobody around is interested in anybody’s works except of her or his own. (This is not a result of moving to some new place; but times did change a lot.) So, it looks like I am willing, but do not qualify.
April 3, 2013 at 4:21 pm
This is really a reply to the comment below (https://gowers.wordpress.com/2013/03/20/pierre-deligne-wins-the-2013-abel-prize/#comment-38721) about videos on the Simons Foundation website.
For some reason that comment does not have a “Reply” link, so I was unable to leave a comment there.
The videos themselves are not protected by any form of content protection, in particular plain video URLs are embedded in the text. Surely the Simons Foundation would not do this if they wanted to protect the videos. Compare this with YouTube, which takes active steps to obscure links to video files, and links themselves are dynamically generated (and therefore cannot be distributed to others).
The problem with the Simons Foundation website seems to be that its administrators seem to be certain that everybody has the latest version of Adobe Flash installed, which is false.
If they were aware of this issue,
they would almost certainly
add HTML5 video elements and direct download links.
For the reference, here is the list of direct URLs to the videos referenced in the comment below:
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Highlights_med.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Math_enthusiast.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Math_competitions.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Competition_and_cooperation_in_the_Hungarian_math_community.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Meeting_Paul_Erdos_and_other_mathematicians.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Solving_a_problem_in_graph_theory.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Graph_limit_theory.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Applications_of_graph_limit_theory.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Controversy_over_combinatorics.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_How_combinatorics_became_legitimate.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Connections_between_topology_analysis_and_graph_theory.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_The_current_status_of_combinatorics.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Attending_Oberwolfach_in_the_Communist_era.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Being_IMU_president.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Prizes_administered_by_the_IMU.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_International_commuting_to_work.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_A_mathematically_talented_son.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Favorite_open_problems.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_P_versus_NP_and_the_Riemann_hypothesis.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Is_randomization_more_powerful_than_deterministic_methods.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Short_vectors_in_lattices_Lovasz_Lenstra_Lenstra.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Combinatorial_theory_of_categories.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Regularity_lemma.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Probabilistically_checkable_proofs_and_hardness_of_approximation.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Writing_books.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_How_mathematics_develops.mp4" /]
[video src="http://simonsfoundation.s3.amazonaws.com/jwplayer/Lovasz/Lovasz_Changes_in_the_Hungarian_math_education_system.mp4" /]
This list was prepared using the following tiny Unix script called simons:
#!/bin/sh
curl -s -S $1 | grep -o “mp4:jwplayer.*mp4” | grep -v /tiny/ | sed s@^mp4:@http://simonsfoundation.s3.amazonaws.com/@ | uniq
For example, the above list was printed by the command
./simons https://simonsfoundation.org/science_lives_video/laszlo-lovasz/
April 4, 2013 at 2:01 am
Dear Dmitri,
Thanks a lot. I wrote a detailed reply to you, but it is hardly related to the discussion here. Please, read it at Simons’s video protection, youtube.com, etc.
March 28, 2013 at 6:00 pm |
Sowa, we all know who you are by now. What was the point of teasing Emmanuel your identity?
March 29, 2013 at 3:13 am
Oh yeah even Ivan Dub knows!
March 29, 2013 at 1:08 am |
I, for one, don’t know who sowa is, nor do I know who Funny Valentine is, and have made no effort to find out. For that matter, there are probably plenty of people who don’t know who “JSE” is, though obviously I make no real effort to be anonymous when I comment on blogs. Why not keep the discussion separate from our real names?
March 30, 2013 at 8:01 pm |
wow, what a saga. found this from GKs blog and his unelaborated ref to the “two cultures in math”. huh? skimmed thru most of the comments & can only say….
wow! sowa is quite the verging-on-narcissistic mathematical troll, enjoying seeing his reflection in all the posted comments. a maybe very dangerous one in particular because he is so well educated, but nevertheless, clearly a troll! it must be an extraordinary accomplishment to achieve such a large flamewar among the worlds most elite mathematicians. congratulations!
found gowers paper on the two cultures & found it interesting/engaging & well written. gowers is a rare mathematician who can write lucidly about auxilary and peripheral topics in mathematics which are sometimes the most interesting.
just wanted add maybe a little fuel to the fire to say that more evidence for the difference of the two cultures comes from a new interview of lovasz on the simons web site, interviewed by noga alon. he talks about the resistance to [rise of] combinatorics by the larger or well established math community. he names a name or two and declines naming some names. [some guilty shall remain nameless].
to me this is all just an application of kuhnian social theory to a technical field. has anyone heard of kuhn? combinatorics is clearly a mathematical example of a kuhnian paradigm shift. it doesnt replace old theory [in this sense it is not precisely kuhnian] but its a new way of looking at mathematics. it will live on.
it has particular relevance in computer science. that alone will ensure its “claim to fame” and will live and endure for the ages. and computer science is tipping the balance toward the problem solvers, at least until we can find a unifying theory!
viva la revolution!
oh & speaking of combinatorics, heres my hot-off-the-[word]press ink-still-fresh tribute to erdos100, brilliant contrarian
March 31, 2013 at 8:55 am
I would like to comment only on “kuhnian paradigm shift”.
As it often happens, my reply is quite long. I posted it as
Combinatorics is not a new way of looking at mathematics.
April 1, 2013 at 5:05 pm
yikes! did not expect a reply. this debate has clearly gotten completely out of control! just want to correct my statement above, lovasz was interviewed by avi widgerson, not noga alon, oops. here is the video. would be interested in others pov on the schism on combinatorics he describes in the “behind-the-scenes” interview.
April 3, 2013 at 5:50 am
About a year and a half ago I tried to watch a video at that site. I will not even try this untill Simons foundation removes the protection of videos preventing their download by standard tools.
I cannot understand why a charitable foundation devoted, in particular, to the dissemination of mathematical knowledge, protects this content as vigorously as, say, Time-Warner.
Why this stuff is not on the youtube.com, after all? Aren’t they interested in some publicity?
I was told in early 2012 that this is, most likely, the result of a mistake by the webmaster or somebody like this, this wasn’t the intention, and the protection will be lifted as soon as possible. It seems that more than a year is not enough.
Very likely it would be illegal in this country even to ask you if you know how to bypass the protection. It would be defintely illegal to tell how to do this.
March 30, 2013 at 8:15 pm |
Prof. Tao wrote:
“This type of result is much closer in spirit to what comes out of what Grothendieck called the “arid steppes” of analysis (such as Fourier analysis): analysis tells us that when analysing the Fourier coefficients of an arbitrary function, then it is occasionally possible to have a single large Fourier coefficient (which, in this context, is the structure playing the role of an integer point on a high genus curve), but this type of structure tends to repel other such structures, so that a given function cannot have too many large Fourier coefficients at once. To encapsulate this fundamental antistructure phenomenon one needs tools from analysis (in this case, things like the Plancherel identity or Bessel’s inequality) than from the structured side of mathematics.”
I like this view of equilibrium between attractive and repulsive sources in a large hybrid mathematical object but I am not sure that analysis is mandatory to express or approach what you call the “anti-structure”. I believe that algebra can build concepts expressing these constraints as nicely as we currently use it/her to express the “crystalline” parts. I agree this is not currently done or mature.
March 31, 2013 at 1:24 pm |
For too long, mathematics was damaged by the prejudice that the only good mathematics is that which pertains to algebraic geometry and topology. Important work in any another area was only deemed worthwhile in how it related to these two areas (most evidently in dynamical systems, and number theory). Fortunately there has been less of this thinking in the last twenty years (arguably the watershed came when Bourgain was denied his Fields’ medal in 1990, due to the extreme prejudice of some members of the committee). Sowa is a throwback to this sad time.
What angers me is his attempt to judge the quality of the work of Gowers and Szemeredi, based on what Sowa’s friends, tangentially connected to this kind of analysis, have said. People in “hard” analysis knew for many years that Szemeredi’s works on k-term arithmetic progressions, regularity, and understanding the spectrum of large Fourier coefficients was far ahead of his time, and it would need extraordinary minds to go beyond him. (Moreover it is not so easy to read Szemeredi’s work, nor to get the big picture from what he writes.) Sowa makes several judgmental remarks on Szemeredi’s work, its influence and its relation to Furstenberg’s work — it sounds like he has some basic knowledge of the area but does this entitle him to make broad sweeping statements as to its value, often justifying this by the opinions of his super-brilliant, yet anonymous, friends?
It is only in the last decade or so that several great mathematicians, starting with Gowers’ far-sighted (and very readable) work, have gone way beyond Szemeredi. Gowers’ work is “seminal”, with his extraordinary development of higher norms, providing the springboard for so much of what was to come (and is still coming).
These developments now affect many areas in mathematics — in fact the story of how big questions in different areas are being tied together by these techniques (and sometimes resolved) is quite reminiscent of the similarly shocking effect that Groethendieck had on algebraic geometry and topology fifty years ago. To act as if all these (more analytic) areas are irrelevant to what is interesting in mathematics, is self-aggrandizing and impressively ignorant. Moreover to write “expanders and growth of groups (in various versions) go back to the works of Margulis, Milnor and Gromov” as if to dismiss the recent brilliant developments, shows no appreciation for the depth and subtlety of the great advances that have been made recently by these new techniques (and I very much doubt that such brilliant minds as Margulis, Milnor and Gromov would be so dismissive).
Sowa writes to Gowers: “you are the main and for few years the only promoter of E. Szemerédi work during the last 15 years (earlier were none)” Perhaps Sowa has heard of Pal Erdos? Erdos always referred to “the great Szemeredi” in his lectures — I never heard Pal give the title “great” to anyone else. Pal always regarded Szemerédi’s work as the deepest and most important that he knew of. I heard that he had tried hard, many years ago, to get Szemeredi some important prizes. As to his recent prizes. The first was the Steele prize, just five (not 15) years ago! Actually it was me, not Gowers, who nominated Szemeredi. It was a lovely experience — four Fields’ medalists (as well as several other top mathematicians) all expressed great enthusiasm for the nomination, and wrote letters explaining how much Szemeredi’s ideas influenced their own most important work. Armed with this information, one might revisit Sowa’s statement:
“there are about 2 orders of magnitude more mathematicians of the level of E. Szemerédi than of level of Milnor or Deligne.”
I have no idea how one can make such a comparison, and such a precise one at that.
These silly prejudices against understanding, or accepting the worth of, the motivations of areas that are not exactly ones own, was never shared by greats like Serre, Birch, Swinnerton-Dyer, Bombieri, Mazur, Wiles,… However, for too long, these deep-rooted prejudices caused number theory to have a wide, artificial split between the arithmetic and the analytic. Fortunately some of the finest work of the last few years has beautiful, penetrating, creative ideas from both sides of the subject — most notably the work of Bhargava, but also of Venkatesh and (this blog contributor) JSE, Poonen and quite a few others. Emerton’s remarks above further outline clearly the argument that this has always been so, if one is open enough to see it. This cross-fertilization is as it should be, and I would encourage participants in this discussion to pay little heed to Sowa’s outdated and divisive prejudices.
Probably we have all found it difficult to judge the importance of a paper, one is refereeing, when it is pertinent to a field just a little distant from one’s own. Members of prize committees have it much tougher. They have to try to judge how penetrating is important work in all of the fields of mathematics, with little real understanding of these fields, but perhaps some intuition of their own, and trusted referees’ letters to read. There is so much great work going on that to criticize a committee for choosing X rather than Y misses the point — there are so many good options that is only important that the choices made are really good ones, as they so often are for the leading awards. None of Langlands, Mazur or Wiles won the Fields’ medal, since there seemed to be better options at those precise moments in time, even if subsequently these seem like opportunities missed. Nonetheless these great mathematicians have all subsequently obtained a lot of deserved recognition, if not that one particular prize.
Finally, there is one remark where Sowa has a point: it is indeed a surprising, yet interesting, decision to have asked Gowers to talk on Milnor’s and Deligne’s works at the Abel proceedings. It is not traditional to ask someone so far afield to present the work of a prize laureate, even someone who is such a brilliant expositor as Gowers. However he presumably was chosen as someone who would give a wonderful and appropriate talk for the audience at hand, and as someone open-minded enough to spend time appreciating the broader aspects of the work and then figuring out how best to present it. It is a ridiculous accusation that Gowers pushed his own candidature for this task — I guess Sowa has no idea as to how such committees work.
March 31, 2013 at 5:26 pm
Interesting. I think Bhargava will win Fields Medal next year together with Avila, Lurie and Green.
May 23, 2013 at 8:06 pm
Probably, I would replace Green with Helfgott now.
March 31, 2013 at 4:39 pm |
Committee that “denied” Bourgain a Fields Medal:
Faddeev (chair)
Atiyah
Bismut
Bombieri
Fefferman
Iwasawa
Lax
Shafarevich
Committee that awarded Bourgain a Fields medal:
Moser (chair)
Deligne
Glimm
Hörmander
Ito
Milnor
Novikov
Seshadri
It is hard to believe the story based on the above lists but if it is true, this brings us back to Deligne, and Milnor, and Atiyah, and Lax, and Abel prize…
March 31, 2013 at 6:05 pm |
@Andrew Granville: “There is so much great work going on that to criticize a committee for choosing X rather than Y misses the point”
“Bourgain was denied his Fields’ medal in 1990, due to the extreme prejudice of some members of the committee”
Which, if either, of these two contradictory positions do you in fact occupy?
April 1, 2013 at 5:30 am |
For too long mathematics was damaged by the prejudice that the only good mathematics is the one which pertains to the Leibniz version of differential and integral calculus. Only such mathematicians as Euler were able to see deeper. I think that there is no need to repeat here the E. Galois story.
Fortunately, during approximately 50 year, 1930ies – 1980ies another way of working in mathematics emerged, in which deep concepts played the main role, and the “executive power” in the sense of Hardy is only marginally important.
Andrew Granville is a throwback to the 18th and19th centuries. In fact, the conceptual methods were always resented by many, if not by majority.
What angers me is A. Granville’s attempt to insult my presumed friends. Are they my friends or not is irrelevant. As I said, I persuaded a colleague to give a series of talks devoted to some results important for Gowers’s work. He is guilty of only one thing: being very enthusiastic and giving several excellent talks. His field is the “hard analysis”. Another “friend” is, apparently, Gowers himself, who gave a series of excellent talks about his work (about arithmetic progressions). The talks of my colleague filled some gaps in Gowers’s lectures (not all, of course). Nothing that I ever said or wrote about Gowers’s results is based on the opinions of these “friends”. They will disagree.
I thought that this is a free country and I am entitled to express my opinions about anything. And I have no idea what Granville means by my “actions”. Writing post and comments? I think the only relevant action by me was the choice of the branch of mathematics I would like to work in.
My remark about expanders and growth of group had only one purpose: to show that the purported example of application of recent work in this kind of combinatorics to the conceptual mathematics is not an example, and the mentioned paper does not depends on any combinatorial results about expanders.
Of course, I heard of Paul Erdős. Unfortunately, I find his personality much more interesting than his mathematics.
It is nice to know that A. Granville nominated E. Szemerédi for Abel prize (I don’t how this excludes the possibility that T. Gowers nominated him to, but does it matter?).
To made a comparison one needs to be to some extent familiar with the work of compared mathematicians. If T. Gowers can be the member of the committee selecting Fields medalists and have no idea about the work of winners (and then reply “no comment” to a direct question about this in his blog), why I am not allowed to made comparisons of mathematicians whose works I studied? And in contrast T. Gowers I am not awarding any prizes here. Why A. Granville worries so much? If I am wrong, all these comments will have no effect at all. May be he suspects that I right?
Note that “about 2 orders of magnitude more” is not a precise estimate.
It should be quite clear that I have no prejudice against any area of mathematics. I had studied more than one unrelated to my work area. The work of Gowers is the most relevant example. Why would I waste my time on it with such a prejudice?
I believe that the mathematical community should be ashamed for not awarding Fields medal to Langlands, Mazur, and Wiles. And because it is a community of mathematically literate people, the mathematical community should be especially ashamed of the stupid age restriction for the Fields medalists, which gives a huge advantage for people born in the year of Congress over the people who are only 3 weeks too older.
This age restriction inevitably gives an advantage to people with high executive power (they solve some problems relatively quickly) over the people with deep insights (which usually require some non-negligible time to mature). In other words, to the problems-solvers over the theory-builders.
I know about how such committees work only one thing: they work with the greatest secrecy possible. Only rarely I am provided with a deeper insight by a friend or somebody who cannot keep the secrets. I see the only way to deal with undesirable conclusions made on the basis of observing the work of a committee as a black box, or just by its output.
If somebody wants to avoid any conjectures about how these committees arrive at their decisions, there is only one way to go: be open. Conduct meetings of these committees openly, so that everyone willing will be able to be present, listen and make records (but not to interfere). Disclose the procedure of selection of the members of, say, Abel prize and Fields medal committee. Nowadays the winners of Fields medals could be predicted by the composition of the committee only, would it be known beforehand. I predicted that this year Abel prize will be awarded to P. Deligne on the following grounds only: the composition of the committee (Abel prize is more open) and the fact that in 2012 it was awarded to E. Szemerédi. I even wasn’t aware that it is the same committee as in 2012.
April 1, 2013 at 2:10 pm |
Let me clarify what I said about prizes. For the most part there is so much great work that to criticize a committee for choosing X rather than Y misses the point. But, just occasionally, there are obvious winners, like recognizing the extraordinary work of Perelman and of Tao for the Fields medal in 2006, and of Ngo in 2010. The travesty of not awarding the Fields’ medal to Bourgain in 1990 led to the 1994 committee awarding three analysis-type Fields’ medals. Similarly the 2002 committee interpreting the rules so as to exclude the great Oded Schramm had its consequences.
Personally I enjoy conceptual mathematics, but I am not sure where to draw the line between that and the concrete — is class field theory, conceptual or concrete? Maybe a little bit of both? Who judges? I do not subscribe to a fight between conceptual vs concrete. Indeed there is so much beautiful mathematics where one feeds off the other (certainly in modern number theory). I grew up in mathematics with the heavy prejudice of people who were trying to appreciate Groethendieck’s work and insisting that almost everything else is “marginally important” (as Sowa writes) to the real issues. I believe that a lot of damage was done by this (formerly common) prejudice, especially keeping down excellent mathematicians who happened to be proving results not to the taste of those, at that time, “in power”. In the 1980s the Fields medals were awarded exclusively to these prejudices — the people chosen are all fantastic mathematicians, but it would have been sensible to have given one more medal, in both 82 and 86, that recognized great work that is a little further afield.
The Fields’ medals of 1998, 2006 and 2010 reflect a more open view of mathematics, and that is healthy. It certainly encourages people in all areas of mathematics. This was Fields’ goal in making the bequest, in which he wanted to create a world prize to encourage the very best “young mathematicians” and “be an encouragement for further achievement on the part of the recipients and a stimulus to renewed effort on the part of others”. A choice had to be made of how to honour that request. The choice made was not perfect, having a silly cut-off and allowing strange interpretations by different committees (and particularly by IMU officials), but if you look at the list of superb laureates (http://en.wikipedia.org/wiki/Fields_Medal), it is hard to be too critical.
April 1, 2013 at 5:17 pm |
was thinking of/ruminating on this thread & the following quote popped into my head. a very interesting & imho apropos quote by plank, pioneer of the atomic theory revolution [along with einstein], which imho is an early recognition of the concept of kuhnian shifts by a 1sthand participant/visionary:
a bit bleak or dark even, but true! it would be interesting if the ages of the participants of this discussion were known. to some degree it is clearly a case of the old guard vs the new fledgling upstarts. as much as such a dichotomy is possible in math research [which sprawls over the decades]. even the upstarts have been at their craft for decades.
April 3, 2013 at 6:42 am
To vznvzn:
Amazing. You read my post “Combinatorics is not a new way of looking at mathematics” linked to in my reply to another comment of you, made some comments there, not even attempted to contest my opinion, but now you are here saying exactly the same things as before.
It is completely wrong that the Gowers-Hungarian-whatever combinatorics is anything really new. It belongs to a very old tradition, preceding by centuries the work of few French mathematicians which laid foundations for the radical departure from this tradition by Grothendieck. It is Grothendieck approach which is new and revolutionary and still accepted only by a minority of mathematician. Unfortunately for the old tradition, the mathematicians using at least partially the new ideology got so impressive results that for about 32 years most (but not all) Fields medals went to them. The rise of the Hungarian combinatorics in the last decade is a (in a precise not judgmental sense) reactionary attempt to accomplish a Wigner shift of the second kind and return to the pre-Grothendieck times. A Wigner shift is similar to what you are taking about, but different and makes much more sense in mathematics. It means abandoning a line of research and moving to another one without incorporating the results of the first into the second.
As of your well known quote, the proponents of pre-Grothendieck mathematics never died out, they always were the majority.
And, what one can expect when somebody has no idea how to respond on substantive grounds? Of course, one should expect an interest in the personal details of the opponents.
Let me tell you that only very few mathematicians will risk to disagree in public with any position supported by a mathematician of such stature in the community as T. Gowers even under a nickname. If not now, then after another such discussion everybody interested will know who sowa in the real world is. Entering the profession of a mathematician is a very hard task. Nowadays it usually requires about 20 years of being uncertain about your future. It is understandable that only few will take any risks during these 20 years. These few usally will indeed have some reasons to speak; the reasons which are hardly understandable by others.
Look, how many people ever rejected a million dollar prize not under duress? To the best of my knowledge, before Grisha Perelman only Jean-Paul Sartre did. But for Grisha the integrity turned out to be much more important than a million, and nobody critically depends on him in terms of money.
April 5, 2013 at 12:27 am |
I take the opportunity that this post has turned into a “two cultures discussion area”. In the the two cultures essay, Tim Gowers writes:
> I have occasionally heard mathematicians on the theory side complain of a problem that it has been attacked with all the known tools, but that a stubborn core remains which is “essentially combinatorics”.
I’m interested in examples of such problems, could anyone please list some?
April 5, 2013 at 12:33 pm
The fundamental lemma (conjectured by Langlands and Shelstad, proved by Ngo), was often described by Langlands as a combinatorial lemma. It was ultimately solved *not* by mastering the combinatorics, but by finding a sufficiently rich theoretical setting in which it could be proved. (As a very rough analogy for how Ngo approached it: imagine you had a difficult problem about nilpotent matrices that you wanted to solve — you could imagine that such a problem could be reduced to combinatorics, because describing Jordan blocks for nilpotent matrices is closely related to partitions. But imagine that instead of trying to work out this combinatorics (which in the actual case of the fundamental lemma had proved pretty intractable), you found a way to enlarge the problem so that it was expressed for all matrices, not just nilpotent ones, in such a way that it was a “continuous function” of the matrix. You could then use the fact that diagonalizable matrices are dense in all matrices to instead try to solve it for diagonalizable matrices instead, which are combinatorially much simpler (just a list of eigenvalues — no partitions any more). )
April 7, 2013 at 1:48 pm
Dear Matthew, This is very interesting. I don’t know what is the “combinatorial” aspects of the fundamental lemma (I will certainly be interested to know what are the combinatorial objects there), but I remember comming accross as a graduate student (in combinatorics) of a Can. J paper by Langlands with a section starting like that
4. Combinatorics. The preparations over, we come now, with sighs of relief from reader and author, to the amusing part of the paper. The combinatorial facts to be verified turn out to be statements about a simple type of tree, the Bruhats-Tits buildings for SL{2). They may well be familiar to combinatorialists, but a cursory glance at the standard texts yielded nothing of help.
At the time, the “combinatorics” looked completely unfamiliar and I never returned to check what it was. (But also here I will be curious to learn.)
On another matter, maybe I am wrong, but my reading of Tim’s two cultures paper at the time was actually as a clear pro-conceptual and not as an anti-conceptual essay.
April 8, 2013 at 12:02 pm
Dear Gil,
This will be a very cursory reply for now, due to time constraints.
For now, I just wanted to remark that the combinatorics in the paper you refer to is a particular case of the fundamental lemma (although not obviously so, even to Langlands; Kottwitz is the one who pointed this out — see the discussion on p.5 of this note.
Regards,
Matthew
April 8, 2013 at 3:40 pm
I blundered in the html for the link in my comment; here it is in plain text:
Click to access sscomments-ps.pdf
April 10, 2013 at 5:51 am
Thanks, Matthew! (Who wrote the note?)
April 10, 2013 at 11:19 pm
Dear Gil,
Langlands wrote it, but some 15 or so years after the original paper
that you referred to appeared. (I think maybe on the occasion of
the reprinting of the paper in some kind of anniversary volume.)
June 21, 2013 at 7:39 am |
I don’t know about your mathematics, or who you are, but the level of your English expression can and should be improved!
June 21, 2013 at 8:01 am |
Paul Erdos once said to me concerning Szemeredi’s work “It is on the boundary of human intelligence”.
As for “Sowa”. Is this a pseudonym? If so, can anyone shed any light on who this person is? His/her English is so poor, it must give clues at least to the first language of the writer.
August 30, 2013 at 11:35 am
@Mike Hirschhorn:
While sowa’s English may be locally imperfect, in a global sense his communication is excellent. He writes very carefully. In any case, attacking the quality of someone’s English seems a poor way of disputing his arguments. Although I have little direct contact with that world, it seems obvious that his English is that of a native speaker of Russian, or some related language.
As for the substance of your post – it seems ridiculous to respond to Sowa’s critique with a laudatory quote from an authority figure, and the more so when the authority figure is the paragon of what Sowa has called Hungarian combinatorics.
September 16, 2013 at 2:20 am |
While it pales beside the passionate arguments above I wanted to mention a small point of precision: Weil regarded cohomology as an analogy and motivation for his conjecture but was not convinced such a cohomology would exist. Grothendieck says so in Recoltes et Semailles page 840, and Serre has said so to me in e-mail (with permission to publish the statement). Weil did not join in pursuing such a cohomology.
September 16, 2013 at 11:13 am
Many thanks: the point may be small but I find it very interesting (and therefore not so small after all).
August 3, 2014 at 12:40 am |
Wow, just came across this stream. I really respect your dedication to open discussion, Tomothy Gowers. Commendable.
November 26, 2014 at 7:19 pm |
gowers has not yet remarked on grothendieck passing. googled this blog and this page seems to have the most mentions of grothendieck (~14), although none by gowers, and all in the comments. here is some tribute with some tie-in to gowers essay “the two cultures of mathematics”. many links, top obituaries etc., and (full disclosure) a so-called “crank” angle.
Grothendieck 1928-2014 — Genius or Crank? BOTH. hope/ encourage gowers to comment/ blog on grothendieck at some pt!
November 26, 2014 at 9:26 pm
I tend to comment on things only if I judge that what I have to say is sufficiently personal, informed, or different from what others have said. In the case of Grothendieck’s passing, that doesn’t apply. I admire him greatly, but from a position of considerable ignorance, so would recommend reading the many obituaries and blog posts that others, who know what they are talking about, have written.
January 26, 2015 at 4:17 pm |
[…] a vulnerable admission in excellent company. Gowers quickly responds! “I admire him greatly, but from a position of considerable […]
February 2, 2021 at 6:59 pm |
Great personal
Pierre Deligne wins the 2013 Abel Prize | Gowers's Weblog
September 16, 2022 at 9:55 pm |
[…] Prime Number Theorem – hardly an achievement of Number Theory itself, if you ask me, *wink wink*. Crucified his ‘motivation to roll the wheel of Geometry again to begin with’ again. […]
December 8, 2023 at 11:26 am |
현금바둑이
Pierre Deligne wins the 2013 Abel Prize | Gowers's Weblog